|
According to classical physics, light is pure energy and has no mass. No mass implies no momentum since the classical definition of momentum is mass times velocity, p = mv. But the wave-particle duality for light allows photons to have no mass but still have momentum. 
 |
It is a common misconception that the Crooke’s radiometer (illustrated at right) demonstrates that light has momentum. Inside the radiometer’s glass shell is a rotating spinner that usually has four vanes; each vane has a black side and a white side. When the radiometer is exposed to sunlight it spins with the white sides leading and the black sides trailing. The black sides absorb light, while the white sides reflect light. If the spinning were due to momentum effects of the light, then the radiometer should spin the other way around—because the white sides cause the photons to change their momentum twice as much as the black sides. Furthermore, the radiometer does not spin if the interior is a perfect vacuum, so the gas inside plays an important role.
Instead, the most likely explanation for why it spins the way it does is that, when the radiometer is illuminated, the black side becomes slightly hotter than the white side. The temperature difference causes a flow of gas from the cold white side to the hotter black side. The pressure difference caused by the gas flow then causes the motion, not unlike an airfoil. 
|
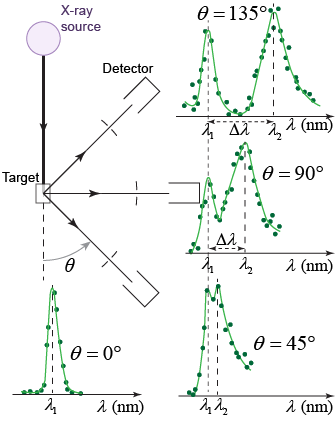In 1923, Arthur Compton conducted a series of experiments on light in which he directed x-rays to hit a graphite target. Compton measured the scattered x-rays at various angles. Although the incident x-rays had a single wavelength, at any given angle Compton found the reflected light to have two different wavelengths. One of the two wavelengths was the incident wavelength λ1, while the other was a slightly longer wavelength λ2. The longer wavelength radiation implied that the energy of this second reflection was lower. Compton explained the shift in energy by postulating that the incident radiation was not a wave but rather a collection of particles called photons, each with energy E = hf.
|
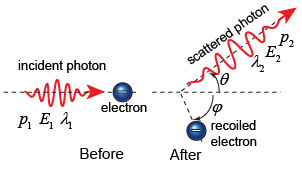Compton deduced that the shift in frequency came from an elastic collision between a photon and an electron in a carbon atom in the graphite. Compton calculated the energy of the scattered photon by applying the laws of energy and momentum conservation:
|
| momentum before | = | momentum after | | and | | | energy before | = | energy after |
|
The collision between a photon and a stationary electron can be solved by considering both the energy and momentum conservation laws. However, since the photon moves at the speed of light and the recoiling electron also moves with very high speed, we must use the relativistic equations for energy and momentum.
The momentum must be conserved along the horizontal and the vertical axes. Momentum conservation along the horizontal axis gives where p is the momentum of the recoiling electron after the collision. Momentum conservation along the vertical axis gives Energy conservation gives us Note that we have written the total energy of the system, including the rest energy of the electron, mec2.
Since E = hf and p = h/λ the shift in wavelength, Δλ, also called the Compton shift, is The quantity h/mec = 2.43×10−12 m and so the shift Δλ is
|
The difference Δλ between the scattered wavelength λ2 and the incident wavelength λ1 increases as the detector angle increases. This result cannot be explained by the wave theory of light. Compton concluded that the photon “carries with it directed momentum as well as energy.” Photons of light conserve energy and momentum in their interactions, much like particles. A complete description of radiation has both wave aspects, to explain interference and diffraction, and particle aspects, to explain how photons interact with matter.
|
|
The interaction between photons and matter is an elastic-type collision. In fact, the scattered wavelength and the observed shift in wavelength λ results from collisions between the photons and the “free” electrons in the target. When a photon interacts with an electron that is tightly bound to the atom and there is not enough energy to eject the electron from the atom, the collision then occurs between the photon and the entire atom. Since the mass of the atom is much larger than the mass of the electron, the scattered photon wavelength during this photon–atom collision will be very close to the wavelength of the incident photon. This is the reason why the peak at λ1 is present at every detector angle.
|
| |
|
