|
Classical physics, such as Newton’s laws, accurately describes the macroscopic world. Quantum theory describes the microscopic world. How do these two very different theories match up? The answer is not just philosophical. There cannot be a sharp boundary at which things suddenly start behaving “quantumly.” The correspondence principle states that quantum theory must transition smoothly into classical physics when the number of particles, energy, or size of a system becomes large. 
|
The de Broglie wavelength gives an approximate length scale for the transition between classical physics and quantum physics. Quantum effects become increasingly important as the size of a system becomes closer to the de Broglie wavelength of the particle. As an example, calculate the de Broglie wavelength of a 0.1 kg ball moving with a speed of 100 km/hr.
|
| Asked: | the de Broglie wavelength λ | | Given: | m = 100 g, v = 100 km/hr | | Relationships: | λ = h/p, h = 6.63×10−34 J s, p = mv | | Solution: | First convert speed to meters per second: v = (100,000 m)/(3,600 s) = 27.8 m/s Calculate the momentum: p = mv = (0.1 kg)(27.8 m/s) = 2.78 kg m/s and so the wavelength is: λ = (6.63×10−34 J s)/(2.78 kg m/s) = 2.38×10−34 m |
|
|
The quantum wavelength of a baseball is 10−34 m! The typical size of a system including a baseball is at least the size of the ball, which is 1034 times larger than the de Broglie wavelength. The scale of the baseball’s system is so much larger than the de Broglie wavelength that we can completely ignore quantum effects.
|
|
Now consider the wavelength of an electron moving at the same velocity. What is the de Broglie wavelength of the electron? (The electron’s mass is 9.11 × 10−31 kg.)
|
| Asked: | the de Broglie wavelength λ | | Given: | m = 9.11 × 10−31 kg, v = 27.8 m/s | | Relationships: | λ = h/p, h = 6.63×10−34 J s, p = mv | | Solution: | p = mv = (9.11×10−31 kg)(27.8 m/s) = 2.53×10−29 kg m/s
λ = (6.63 ×10−34 J s)/(2.53×10−29 kg m/s) = 2.62×10−5 m |
|
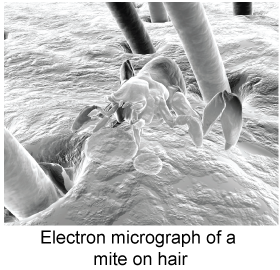The electron’s wavelength is around 10−5 m. This is larger than an atom and comparable to the size of a virus particle. The electron microscope is an instrument that uses electron waves instead of light waves to make very high magnification images, such as this image of a dust mite hiding in the fur of an animal (right). Optical microscopes cannot image anything smaller than the wavelength of visible light. The electron wavelength can be much shorter, allowing the electron microscope to image much smaller objects.
|
Calculate the de Broglie wavelength λ of a neutron having a kinetic energy Ek of 2.0 MeV.
 |
The de Broglie wavelength is given by the equation λ = h/p.
Momentum is given by p = mv and is related to kinetic energy Ek by The mass of the neutron is 1.675×10−27 kg, so the de Broglie wavelength is 
|
