|
|
The hydrogen atom offers the simplest example of emission and absorption because it has only one proton in the nucleus and one electron orbiting around it. Some of the unusual features of the quantum theory were accepted remarkably quickly because of the success of Bohr’s model in matching the observed wavelengths of the line spectrum for hydrogen. 
|
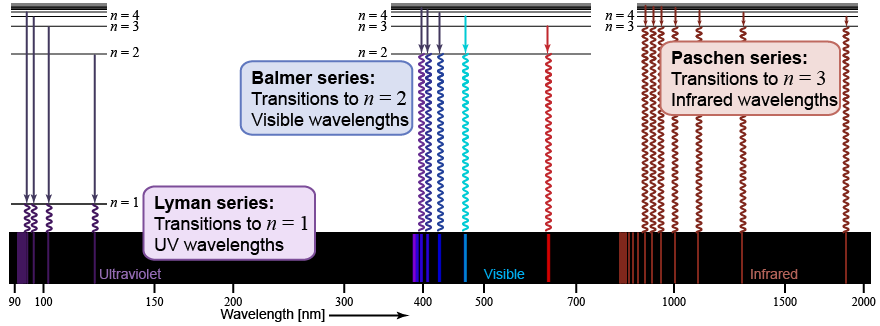
Hydrogen gas emits spectral lines at ultraviolet (UV), visible, and infrared wavelengths. Atomic transitions to and from the n = 1 energy level correspond to wavelengths of light in the ultraviolet and are named the Lyman series. Atomic transitions between the n = 2 energy level and higher n levels correspond to wavelengths of light in the visible and near-UV and are named the Balmer series. Atomic transitions between n = 3 and higher quantum numbers correspond to photon energies in the infrared. The lines corresponding to the Lyman series have the highest energy, followed by the lines of the Balmer series. 
 |
Emission lines of the hydrogen atom in the Balmer series between n = 2 and higher values of n are visible to the human eye. The wavelengths for these atomic transitions were known to Bohr, but explaining them was the challenge of the day. In the Balmer series, the transition from n = 3 to n = 2 corresponds to the emission of a photon at a wavelength of 656 nm, which is red light. That is why hydrogen has an emission line at 656 nm. The transition from n = 4 to n = 2 produces a photon at a wavelength of 486 nm (green-blue light), n = 5 to n = 2 produces 434 nm (blue light), and n = 6 to n = 2 produces 410 nm (violet light). 
|
A hydrogen atom has an electron at an energy level of −5.45×10−19 J. It suddenly makes a transition to the −2.18×10−18 J energy level.
(a) Did it emit or absorb a photon? (b) What is the energy of the photon?
(c) What is the photon’s wavelength?
(d) What part of the electromagnetic spectrum does this wavelength correspond to? | Asked: | (a) whether it emits or absorbs a photon; (b) energy of the photon;
(c) wavelength of the photon; (d) region of the electromagnetic spectrum | | Given: | initial energy level of −5.45×10−19 J, final energy level of −2.18×10−18 J | | Relationships: | Planck equation E = hf, speed of light equation c = fλ | | Solution: | (a) The atom lost energy; therefore, it emitted a photon.
(b) The atom’s change in energy equals the photon’s energy: (c) Substitute the speed of light equation into the Planck equation to get ΔE = hc/λ. Solving for λ gives (d) This wavelength is in the ultraviolet on the figure on page 641. | | Answer: | (a) It emitted the photon; (b) 1.64×10−18 J; (c) 121.7 nm; and
(d) ultraviolet portion of the EM spectrum. | 
 |
If the atom transitions to a higher energy state, then it is gaining energy by absorbing a photon. In this case, ΔE > 0 because the change in energy is the final energy minus the initial energy. Similarly, if the atom transitions to a lower energy state, then it is losing energy by emitting a photon and ΔE < 0. But if you tried putting a negative ΔE into the equation in part (c) above, you might think (incorrectly) that the emitted photon has negative energy. But photons can never have negative energy!
When you calculate the photon energy, as in the solved problem above, always use the absolute value of ΔE. The sign of the difference in energy, ΔE, is telling you whether a photon is absorbed or emitted. The sign is not telling you that the emitted photon has negative energy—that’s just plain impossible! 
|
Which of the following electron transitions in a hydrogen atom would emit visible light? - n = 1 → n = 2
- n = 3 → n = 1
- n = 4 → n = 2
- n = 5 → n = 3
 |
The correct answer is c. When electrons of a hydrogen atom lose energy and transfer to the n = 2 energy level, visible light is released. 
|

