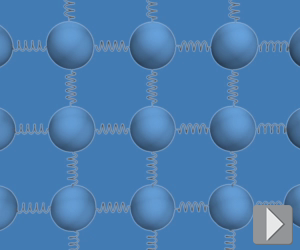|
In a solid, the atoms (or molecules) are close together and interact strongly. Imagine a 3-D array of masses connected by springs that represent the bonds between neighboring atoms. Like actual springs, interatomic bonds store potential energy when extended or compressed away from equilibrium. Because atoms are so close together, the concept of thermal speed is not strictly accurate because thermal energy is shared between kinetic energy and potential energy in bonds. 
|
|
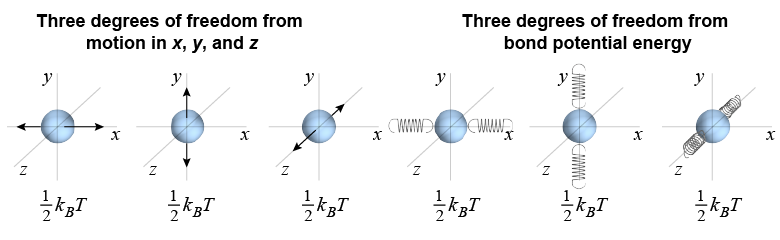
Consider a monatomic solid such as a metal. Kinetic theory still holds that each independent degree of freedom has an energy of ½kBT as long as the temperature is not too low. In addition to the three degrees of freedom from motion in x-, y-, or z-directions, there are three additional degrees of freedom associated with potential energy stored in the bonds (or “springs”) that connect atoms to each other.
|
|
The total thermal energy is therefore 3kBT per particle. If we let the thermal energy for one mole of particles be equal to the heat Q to raise the temperature from absolute zero to temperature T, we get the following result for the specific heat of a monatomic solid:
|
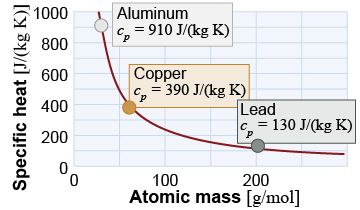This model explains the law of Dulong and Petit, which says that the specific heat of a monatomic solid decreases as the inverse of the atomic mass. The predicted specific heats of aluminum, copper, and lead are 898, 385, and 130 J/(kg K), respectively. These compare well with the actual measured values of 910, 390, and 130 J/(kg K), as shown in the illustration at right.
|
| |
|
