|
Streamlines are drawn parallel to the direction of flow and show how fluids flow around the surfaces of objects such as vehicles and aircraft. Where streamlines are close together, speed is higher. Where streamlines are farther apart, speed is lower. Energy is conserved only along the same streamline, because a streamline tracks the motion of the same specific mass of fluid as it flows. 
|
|
Bernoulli’s equation tells us that along a streamline the variables of height, pressure, and speed are related by energy conservation. If any of these three increases, at least one of the other two must decrease. For example, if the speed of a fluid goes up, the pressure may go down to compensate.
|
|
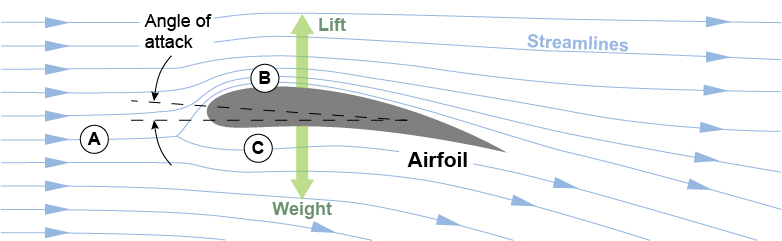
Flight is an important application of fluid flow in which the fluid is air. In order to fly, a plane in motion must generate a vertical lift force at least equal to its weight. The purpose of a wing is to manipulate airflow in a specific way to create and control lift forces. The cross section of a wing has the shape of an airfoil. As shown in the illustration below, the shape of an airfoil and the angle of attack force air along streamline (A) to be divided into two paths: air flowing across the top of the wing (B) and air flowing under the wing (C). During the same time interval, air flowing over the wing must travel a further distance than air flowing under the wing, so the speed of air over the top surface of the wing (B) is higher than the speed below the wing (C). This difference in the speed of the airflow is the key to flying.
|
|
According to Bernoulli’s equation, the sum of energy densities must be equal for both paths that originate along the same streamline at (A). Increasing the speed above the wing therefore means that the pressure on the top surface of the wing is lower than the pressure on the lower surface—since above and below the wing all the other parameters in Bernoulli’s equation (ρ, g, and h) are equal. The difference between the pressures above and below the wing, when multiplied by the area of the wing, generates the lift force. Because the lift force is created by the motion of air over the wing, the force increases as the square of the plane’s speed. That is why planes need to accelerate along a runway until they reach take-off speed. A plane cannot get off the ground until it reaches a speed at which the lift force exceeds the plane’s weight.
 |
To calculate the lift force, assume that two streamlines start very close together (A) and have the same value of pressure and airspeed. Streamline B goes over the top surface of the wing and streamline C goes under the wing. Bernoulli’s equation says that ρgh + ½ρv2 + P must be the same at points B and C since they were the same at point A.
The density of air is about 1.3 kg/m3 and a typical take-off speed is 50 m/s. Under the wing the air slows somewhat, so suppose the average speed is 40 m/s. The airspeed over the leading edge might be 70 m/s. According to Bernoulli's equation, the difference in pressure above and below the wing, PC − PB, is 2,145 N/m2. A plane with a wing surface of 30 m2 would feel a lift force of 2,145 N/m2 × 30 m2 = 64,000 N, enough to lift a 6,600 kg plane weighing more than 14,000 lb!
We have neglected the potential energy term completely. Why? The answer is that when you substitute values the change in this term is negligible compared to the other two. For example, suppose the wing were 0.5 m tall. The change in pressure caused by the change in potential energy is ρgh = (1.3 kg/m3)(9.8 N/kg)(0.5 m) = 6.37 Pa Compared to 2,145 Pa from the change in velocity, this term is completely unimportant.
Note that this calculation gives a very rough estimate because we have assumed that the velocity is constant across the whole top or bottom surface of the wing. In a correct calculation we would need to determine the velocity at every point along the wing surface. The velocity and pressure vary considerably and the net force acting on the wing is not directly upward but tilted rearward, against the motion of the plane. The rearward component is called drag and overcoming drag is the reason aircraft have engines! The drag force is the price you pay for ramming the wing through the air fast enough to generate lift. There are many shapes of airfoils that trade off greater lift at low speed versus drag at high speed. The design of wings and airfoils is part of the field of aerospace engineering and applies not only to aircraft but also to ships moving through water and to cars on the highway. 
|
| |
|
