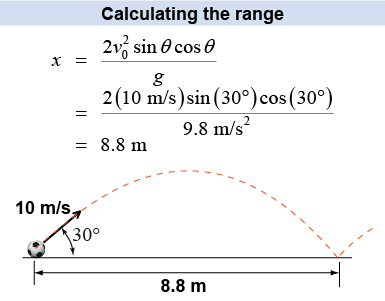|
Now that we have the range equation (6.10), we can calculate how far a soccer ball goes when kicked upward at an angle of 30º with an initial velocity of 10 m/s. If we use the values v0 = 10 m/s and θ = 30º to plug into equation (6.10), then we find that the range of the ball is 8.8 m, which is approximately 30 ft. 
|
Looking at the equation we see that the speed appears as v2, which tells us that the range increases with the square of the speed. If you launch at twice the speed, the ball will go four times as far. Kicking the ball four times faster gets you a factor of 16 increase in range! At speeds much over 10 m/s, air resistance becomes impossible to ignore, so the range equation is not accurate at higher speeds!
 |
The dependence on angle is more complex since there are two terms multiplied together: sin θ × cos θ. The sine is the ratio of the opposite side of the triangle to the hypotenuse. When the angle is zero the opposite side is zero and therefore the sine is also zero. As the angle is approaches 90º the opposite side becomes the same length as the hypotenuse and therefore the sine of 90º is 1. Cosine is the ratio of the adjacent side of the triangle to the hypotenuse and has the opposite behavior. Near 0º the adjacent side of the triangle is the same as the hypotenuse so the cosine of 0º is 1. The cosine of 90º is zero. 
|
|
In the range equation (6.10), the range depends on the initial projection angle θ through the product of sin θ and cos θ. When you multiply sine and cosine together, the combined function is zero when either sine or cosine is zero. That means that the range is zero at 0º (when the ball is kicked along the ground) and at 90º (when kicked straight upward). In the absence of air resistance, the range is maximum at 45°—halfway between 0° and 90°.
|
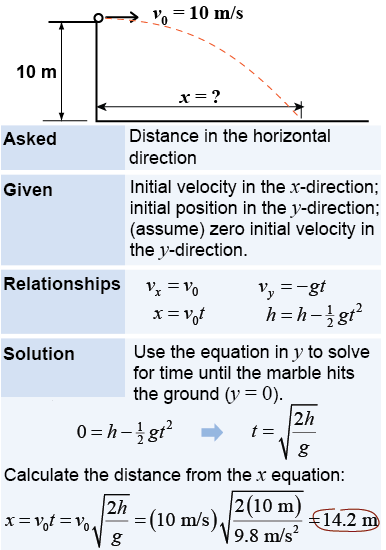Not all projectile problems start with an angle. Suppose you launch a marble horizontally off a cliff at a speed of 10 m/s. If the cliff is 10 m high, how far will the marble travel before it hits the ground?
The first step in the solution is to write down the equations of motion in the x- and y- directions, omitting any terms that are zero. In this problem, x0 = 0 and vy0 = 0. The marble starts at y0 = h with initial velocity vx0 in the x-direction. The y equation for position has only one unknown (time), so solve it for the time. The time is substituted back into the position equation in the x-direction to calculate the answer. The marble travels 14.2 m before it hits the ground.
|
For an initial velocity of 10 m/s, tabulate the range for projection angles 30°, 40°, 45°, 50°, and 60°. What angle produces the maximum range?
 |
Using the range equation (6.10) with v0 = 10 m/s results in the following table: Range for different projection angles Projection angle θ
(degrees) | Range x
(m) |
|---|
| 30 | 8.8 | | 40 | 10.0 | | 45 | 10.2 | | 50 | 10.0 | | 60 | 8.8 | A projection angle of 45° produces the maximum range. 
|
