- A particular service station in Canada sells gasoline for $0.85 per liter. If your tank holds 14 gallons, then what is the cost to fill up?
- Which is longer, 100 miles or 150,000 meters?
- The summit of Mount Everest at 8,848 m is the highest point on the Earth’s surface. If a small airplane can fly no higher than 30,000 ft, can it fly directly over the summit of Mt. Everest?
- Convert the time 3:24:22 to seconds.
- Convert 55 miles per hour into meters per second.
- One cubic yard is equal to how many cubic feet?
- On your 50th birthday, how many seconds have you lived?
- What is the volume of a steel marble that has a mass of 50 g? (The density of steel is 7,800 kg/m3.)
- Which is longer, 10 million seconds or 100 days?
 How long is a 5 mile road in centimeters?
- One mil is 0.001 inch. How many millimeters is one mil?
- One acre is 43,560 square feet. (There are 5,280 feet in a mile and 3.28 feet in a meter.)
- How many square miles is one acre?
- How many square meters is one acre?
- How many square kilometers is one acre?
- Convert 1,500 revolutions per minute (rpm) into revolutions per second.
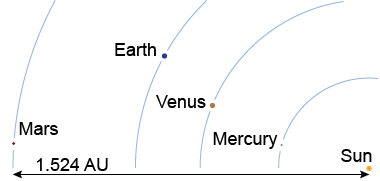
- The average orbital radius of Mars is 1.524 astronomical units (AU). One astronomical unit is the radius of the Earth’s orbit, or 1.5×1011 m. What is the orbital radius of Mars in kilometers?
| | 
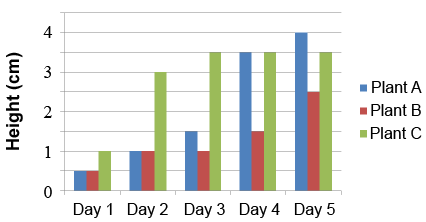
 Measurements were made of the heights of three different plants over five days. The data collected are displayed in the chart above.
- Which plant grew the most over the five days the plants were measured?
- Which plant grew the most between day 1 and day 2?
- How could you infer those conclusions using the bar chart?
- Generate a new bar chart that plots the change in the height of each plant between days, i.e., between days 1 and 2, 2 and 3, 3 and 4, and 4 and 5.
- Which plant grew the most on any single day?
- A bar chart may not be the best way to represent these data. What kind of graph or chart would you use to plot the height of these plants? Explain.
- Convert 293 cubic feet per hour into liters per second.
- Refer to the table to answer the following questions.
Position x
(m) | Speed v
(m/s) |
|---|
| 0.0 | 4.0 | | 5.0 | 3.0 | | 10.0 | 2.0 | | 15.0 | 1.0 | | 20.0 | 0.0 |
- Graph position and speed using speed as the dependent variable.
- Draw a trend line through the data points. What is the slope of your graph? Be sure to use the correct units in your answer.
- Use the graph to estimate the position when the speed is 2.5 m/s.
- Write an equation that is a model to represent the data.
- Use your graph to estimate the speed when the position is 6.8 m.
- Use the equation to estimate the speed when the position is 6.8 m.
- What is the range of values of the dependent variable?
|
