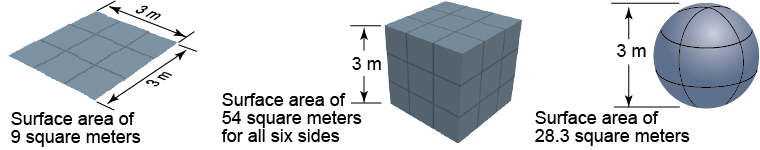
|
The fundamental unit of length appears in other spatial quantities such as surface area and volume. Surface area describes how many square units it takes to completely cover a surface. For example, the top of a 3 m × 3 m board has a surface area of 9 m2. A 3 m cube has a surface area of 54 m2. A sphere with a diameter of 3 m has a surface area of only 28.3 m2, considerably less than a cube of the same dimension. All units of surface area are units of length squared, such as square inches (in2) and square kilometers (km2). 
|
|
|
Volume measures an amount of space in units of length cubed. Examples include cubic meters (m3), cubic centimeters (cm3), and cubic inches (in3). Volume is such an important concept that there are specific units for it, such as liters and gallons. Volume-specific units can always be related to the fundamental length units on which they are based. For example, one liter is defined as a volume of 1000 cm3 and one gallon is 3,780 cm3. For simple geometric shapes, the surface area and volume can be calculated by using formulas such as the examples in the diagram below.
|
|
|
Which has more mass, a block of steel or a block of wood the same size? Both blocks have the same volume but the steel block has more mass. The steel block has more mass because the density of steel is higher than the density of wood. The density of an object measures the concentration of matter in the object’s volume. The units of density are units of mass divided by units of volume, such as kilograms per cubic meter (kg/m3). Air has a low density, typically about 1 kg/m3. Steel has a much higher density of around 7,800 kg/m3.
|
The equation for calculating density is given by equation (2.2). | (2.2) | | | ρ | = | density (kg/m3) | | m | = | mass (kg) | | V | = | volume (m3) |
| Density
|
 |
The lowest density matter you experience every day is probably air at about 1 kg/m3. Water has a density of 1,000 kg/m3. The densest solid you are likely to ever touch is platinum at 21,450 kg/m3. In general, gases are less dense than solids or liquids. The rest of the universe contains a much wider range of density, with the low being near zero in the empty space between galaxies. (The average density of the universe is around 5×10−27 kg/m3, which corresponds to only a few hydrogen atoms per cubic meter. The voids between galaxies are even less dense than that!) The atmosphere of Mars has a density of 0.006 kg/m3, about 1/160th the density of Earth's atmosphere. The core of the Sun has a density of 160,000 kg/m3 and the densest known matter, a neutron star, has a density of 4×1017 kg/m3. 
|
A conical narwhal tusk is 2.0 m long and 4.0 cm wide at the base. What is the volume of the narwhal tusk? - 0.000838 m3
- 0.00335 m3
- 0.00251 m3
- 0.0127 m3
 |
The correct answer is a.
Asked: Volume of the narwhal's tusk
Given: The tusk is a cone, height of the tusk h = 2.0 m, diameter of the tusk d = 4.0 cm
Relationships: 100 cm = 1 m, radius is half of diameter (r = d/2), volume of a cone is V = ⅓πr2h
Solve:
Convert diameter in centimeters to radius in meters: Solve for volume: 
|
This narwhal tusk has a mass of 1.6 kg. What is the density of narwhal tusk? - 0.0251 kg/m3
- 2,980 kg/m3
- 1,900 kg/m3
- 0.00127 kg/m3
|
The correct answer is c.
Asked: Density of the narwhal tusk
Given: Volume of the tusk V = 0.000838 m3 (previous answer), mass of tusk m = 1.6 kg
Relationships: ρ = m/V
Solve:
|
Take a Quiz |
| |
|
