|
Optics is the science and technology of light. One of the most important inventions in optics is the lens. There is a lens in each of your eyes, and lenses are found in cameras, microscopes, telescopes, and projectors. Lenses use curved surfaces to refract light in specific ways. 
|
Lenses
|
|
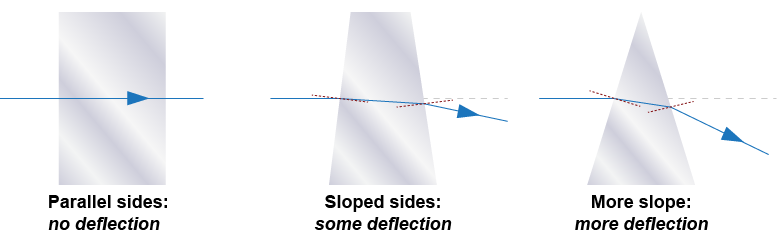
Consider a light ray encountering the three shapes of glass in the diagram below. A flat-sided shape does not deflect the incident ray at all. A slope-sided shape, however, deflects the incident ray and the deflection increases with the slope of the surfaces.
|
|
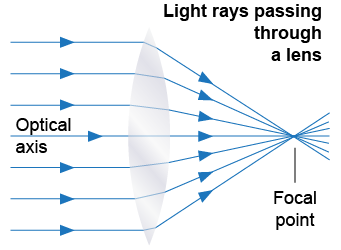In a lens, the surfaces are continuously curved. At the center, on the optical axis, a light ray is not deflected. Light rays that enter the lens parallel to the optical axis are refracted more and more as their distance from the optical axis increases. In a perfect lens, the surface is curved in just the right way so as to bend all the light rays—no matter what their distance from the optical axis—to meet at the focal point.
|
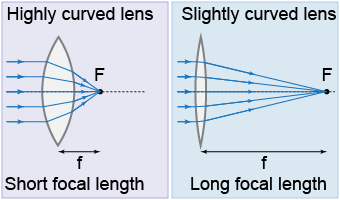The focal length of a lens is the distance from the center of the lens to the focal point. The focal length depends on the curvature of the surfaces and the index of refraction of the lens material. A thick lens with strongly curved surfaces deflects light rays more and therefore has a shorter focal length than a thin lens with more gradual curves. A lens made of material with a high n has a shorter focal length than a lens made of a low-n material.
 |
How does the focal length of a lens depend on the curvature of the two surfaces of the lens and the index of refraction? In the case of a spherical mirror, the focal length is one-half of the radius of curvature of the mirror. Biconvex lenses are more complicated because they have two surfaces, not one, and refraction is occurring within the lens. If we label the two radii of curvature as R1 and R1, and if we assume that the lens thickness is thin, then an approximation of the lensmaker's formula tells us the focal length of the lens: | | | f | = | focal length of lens (m) | | n | = | index of refraction of lens material | | R1 | = | radius of curvature for lens surface 1 (m) | | R2 | = | radius of curvature for lens surface 2 (m) |
| Lensmaker’s formula
thin lens approximation |
Note that a convex surface in the direction light is traveling has positive R, so R1 is positive and R2 is negative for a biconvex lens (because the second surface is concave when looking at it from left to right). The focal length of the lens is therefore inversely proportional to (n − 1). Its dependence on the radii of curvature is more complicated. For a symmetrical biconvex lens, where both surfaces have the same radius of curvature R, the equation reduces to f = R/[2(n − 1)] making f proportional to R. 
|
|
The diameter of a lens determines how much light is collected at the focal point. A large-diameter lens collects more light than a small-diameter lens. That is why expensive cameras have larger lenses. It takes a minimum amount of light to register an image on the electronic detector in a digital camera. A large lens collects enough light for the camera to be able to take a good picture even in dim light, such as indoors. Cellphone cameras can only take good pictures in bright light because they have much smaller lenses.
|
