|
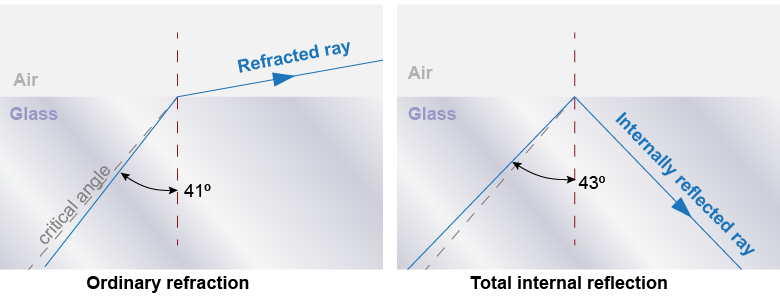
When light passes from air into glass, there is usually some reflection and some transmission. The transmitted light is the refracted ray and it bends toward the normal according to Snell’s law. When light passes from glass into air, however, something different may happen. When the angle of incidence is 41° the refracted ray bends 80° away from the normal. When the angle of incidence is 43° there is no refracted ray at all! The incident ray is reflected back into the glass and no light is transmitted into the air! This phenomenon is known as total internal reflection. 
|
|
The critical angle is the angle at which a refracted ray bends 90° away from the normal. When the angle of incidence is greater than the critical angle an incident ray is totally reflected and there is no refracted ray. The critical angle depends on the ratio of the indices of refraction on either side of the boundary. Equation (21.2) tells us that the sine of the critical angle is the lower refractive index divided by the higher refractive index. 
 |
How are Snell’s law and the equation for critical angle related? Total internal reflection occurs when the refracted light ray is so far from the normal that it is coincident with the boundary between the two media. This occurs at a refracted angle θr ≥ 90°. Remember that sin 90° = 1, so substitute sin θr = 1 into Snell’s law to get: | |

|
| (21.2) | | | θc | = | critical angle (degrees) | | nr | = | index of refraction for refracted medium | | ni | = | index of refraction for incident medium |
| Critical angle for
internal reflection
|
|
An optical fiber is a pipe for light rays that works on the principle of total internal reflection. Consider sending light through a glass rod at an angle of incidence greater than the critical angle (with respect to the wall of the rod, not the end). At every point, light is totally internally reflected off the boundary between glass and air and bounces back into the glass. If the glass rod is made very thin, it becomes flexible, but still traps light by total internal reflection. Optical fiber cables form the backbone of all high-speed telecommunications lines, spanning continents and oceans carrying voice and network data. In another application, a bundle of optical fibers is the core of a flexible image pipe in which each fiber transmits one dot of the image. 
|
What is the critical angle for light passing from glass (n = 1.50) to air (n = 1.00)? | Asked: | critical angle θc going from glass to air | | Given: | incident material (glass) ni = 1.50, refracted material (air) nr = 1.00 | | Relationships: | sin θc = nr / ni | | Solution: | | |
| | Answer: | θc = 41.8°. Compare this angle to the diagram at the top of the page! | 
|
Light traveling from an unknown material to air is completely internally reflected at an angle of 25.0°. Using the table on the previous page, identify the material.
 |
The material is diamond.
Asked: to identify the incident medium
Given: refracted medium (air) with nr = 1.00; total internal reflection at θ = 25.0°
Relationships: sin θc = nr⁄ni
Solve: The incident material can be identified by its index of refraction, ni. Use Rearrange and solve for ni, using the given angle as the critical angle: The index of refraction is at least 2.36. The only material in the table with an index of refraction higher than 2.36 is diamond with an index of refraction of 2.42. 
|
When light is traveling from a material into air, what is the relationship between that material’s index of refraction and the critical angle? - As the index of refraction increases, the critical angle increases.
- As the index of refraction increases, the critical angle decreases.
- Index of refraction and critical angle are unrelated.
- There is not enough information to answer the question.
 |
The correct answer is b. Just taking a few values from the table of materials and indexes of refraction will reveal the correlation: 
|

