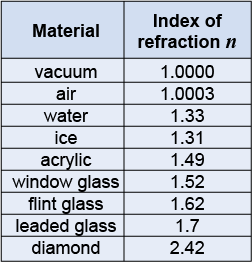|
The angle by which a light ray is refracted toward or away from the normal depends on the index of refraction on both sides of the boundary. The higher the difference between the two indices of refraction on either side of the boundary, the greater the refraction. Among common materials, the index of refraction varies from 1 (air) to 2.42 (diamond). The table on the right lists some typical values. The greatest difference we would normally see occurs between air and diamond. In part, this explains the characteristic “sparkle” of a polished diamond. 
|
|
Snell’s law of refraction relates the angles of the incident and refracted rays to the index of refraction on both sides of the refracting boundary. The formula says that the product of the index of refraction multiplied by the sine of the angle is the same on both the incident and refracting sides of the boundary. In equation (21.1) for Snell’s law of refraction, , ni and θi refer to the medium containing the incident ray. Similarly, nr and θr refer to the medium containing the refracted ray.
|
| (21.1) | | | ni | = | index of refraction, incident medium | | nr | = | index of refraction, refracted medium | | θi | = | angle of incidence (degrees) | | θr | = | angle of refraction (degrees) |
| Snell’s law
of refraction
|
|
|
When using Snell’s law, always remember to reference the angles of incidence and refraction to the normal, not to the boundary interface between the materials! The solved problem below illustrates what we mean. The sine of an angle is a mathematical function that varies from zero to one as the angle changes from 0° to 90°. In standard mathematical notation, sin(0°) = 0 and sin(90°) = 1.
|
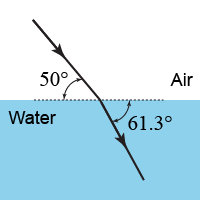A light ray traveling from air (nair = 1.0) into the ocean is deflected, according to the figure at right. What is the index of refraction of the water? | Asked: | index of refraction of water, nw | | Given: | index of refraction of air, na = 1.0;
figure showing incident and refracted angles | | Relationships: | Snell’s law: | | Solution: | From the figure, recognize that the incident angle is θi = 90° − 50° = 40° and the refracted angle is θr = 90° − 61.3° = 28.7° Solve for the index of refraction of water: | | Answer: | nw = 1.34.
(Note that this is the value for saltwater!) |
|
For light traveling through air and then entering another medium, which material bends light more, water or diamond?
 |
Diamond bends light more than water. As shown in the table at the top of the page, the index of refraction of diamond is higher than that of water, which means that diamond refracts or bends light more than water does. 
|
|
What would happen to a light ray that enters a parallelogram prism with parallel sides at an angle?
|
|
The ray would refract when it entered the glass but would refract back when it exited. This means the ray would exit along a path offset from but parallel to its initial path, even though it was refracted in between.
|
