|
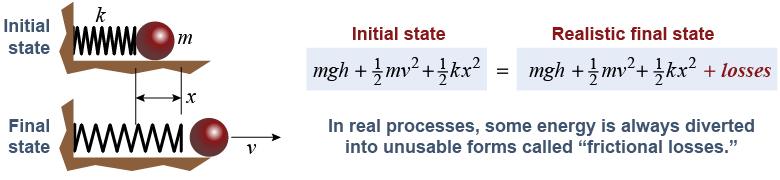
In our initial treatment of energy conservation we assumed 100% conversion of mechanical energy among various forms. In reality, every transformation of energy diverts some energy into heat, wear, or other forms gathered into the catch-all basket of frictional losses. When a system has changed, any energy diverted into frictional losses reduces the energy available for mechanical forms, such as kinetic, gravitational potential, and elastic potential energy. 
|
|
The law of conservation of energy states that energy can be neither created nor destroyed, only transformed. If we counted every form of energy, then the law of energy conservation would be perfectly obeyed. Unfortunately, the forms of energy that become “frictional losses” are not reflected in the observable properties of the system, such as speed, height, or length. Strictly speaking, if only observable forms of energy, such as kinetic energy, are counted then the system is open and frictional energy crosses the boundary, out of the system. That is why we loosely call frictional energy “losses.” 
|
The closed system is always an approximation in the macroscopic world. Nonetheless, the approximation is extraordinarily useful for analyzing the physics of an incredible variety of natural and technological systems! If the frictional losses are small—often less than 10%—then the approximation can be very good. The predictions of a model based on closed-system energy conservation often will accurately reflect real experiments. Even when friction is present and important, the ideal, frictionless case provides a “best-case” estimate of changes in a system. 
|
This is an important point: Energy conservation sets an upper limit on what changes are possible. If we solve the spring problem on page 282, then we find that frictionless ideal energy conservation predicts a speed of 5.6 m/s. 
|

|
Any frictional losses would reduce the kinetic energy of the ball in the final state of the system. Therefore, 5.6 m/s is the maximum possible speed the ball could have if friction could be reduced to insignificance. When a problem on a physics exam asks for the “maximum” speed or height, the problem is actually asking you to approximate the system as ideal and frictionless. 
|
| |
|

