|
A spring allows for a third form of energy: elastic potential energy. In problems with springs, the total energy has three terms: one each for gravitational potential energy, elastic potential energy, and kinetic energy. 
|
|
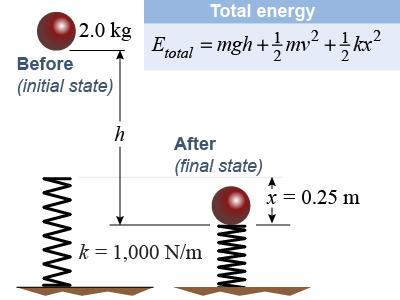
Consider a 2.0 kg ball that drops onto a vertical spring with a spring constant k of 1,000 N/m. From what height did the ball drop if the spring compresses by 25 cm?
| 
|
|
To solve the problem we follow the same three steps. First, name the variables and write down the total energy of the system in the initial and final states, before and after the change. Then eliminate terms that are zero. The remaining equation contains the solution.
|
|
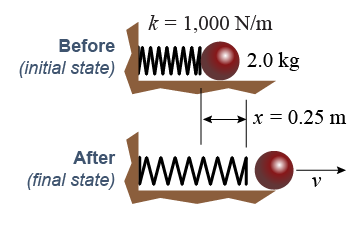A horizontal spring is used to launch a 2.0 kg ball. The spring is compressed by 0.25 m and has a spring constant k of 1,000 N/m. What is the maximum speed of the ball? | Asked: | speed v | | Given: | k = 1,000 N/m; x = 0.25 m;
m = 2.0 kg | | Relationships: | total mechanical energy:
Etotal = mgh + ½mv2 + ½kx2 | | Solution: | |
|
|
|
|
