|
In all free fall problems you will need to be careful regarding the sign of g. The acceleration of gravity is a constant g = 9.8 m/s2 downward. The value of g, however, can be positive or negative depending on how you define your coordinates. - If up is defined to be positive, then g = −9.8 m/s2.
- If down is defined to be positive, then g = +9.8 m/s2.
|
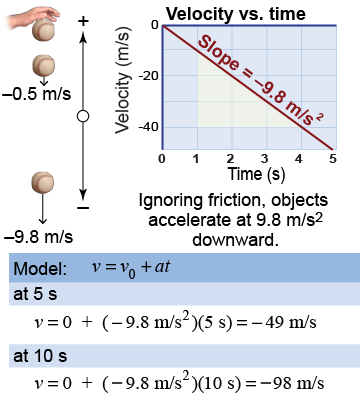Consider a ball that is dropped straight down from rest. Ignoring air friction, gravity causes a constant downward acceleration of −9.8 m/s2. Therefore, 9.8 m/s is subtracted from the ball’s velocity each second. After 5 s of falling the ball’s velocity is −49 m/s. After 10 s the velocity is −98 m/s, which is over 200 miles per hour! Notice that, in this example, negative acceleration causes the speed to increase, because both acceleration and velocity are in the same direction. The speed increased even as the velocity became more negative!
|
|
In all free fall problems the first step is to choose coordinates and assign the value of g to be positive or negative. Then rewrite the equations of motion, eliminate terms that are zero, and substitute g for a. Finally, work out and execute a strategy for getting an answer.
|
|
|
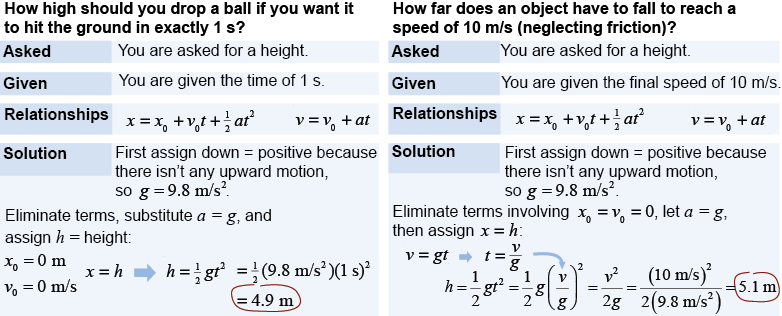
In both example problems, we assume zero initial position and zero initial velocity. Unless a problem explicitly gives you an initial velocity or height, you should do the same. After eliminating terms involving x0 and v0 we see that the first problem has only one unknown, h, and therefore can be solved using only the position equation. The second problem requires both equations since there are two unknowns: v and t.
|
| |
|
