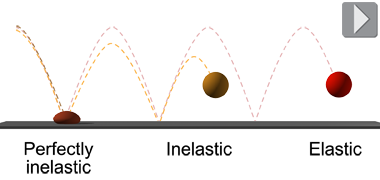|
Collisions happen in many ways: A cue ball strikes other pool balls, a hiker brushes past branches, a baseball player strikes a fastball, one car rear-ends another car, the space shuttle docks with the space station, or a baker pounds bread dough. People do not usually refer to all of these events as collisions, but in all these cases one object comes in contact with another object. In physics, a collision is any such interaction that causes one or more objects to change its velocity. As you will learn in this section, all collisions can be categorized as elastic, inelastic, or perfectly inelastic. In the illustration at right, you can describe qualitatively the outcome of each type of collision based on how high the ball bounces. How can we describe collisions quantitatively using physics? 
|
Conservation of momentum in collisions
|
|
As we learned on page 313, momentum is always conserved for a closed system. The law of conservation of momentum applies to all different kinds of collisions, whether the two objects bounce off each other or stick together.
|
|
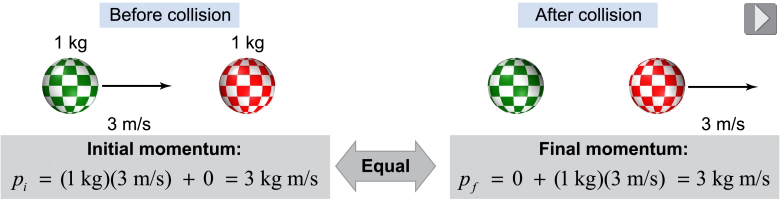
In the illustration above, two balls with masses of 1 kg collide. The red ball is stationary before the collision, and the green ball is stationary after the collision. How can we check that momentum is conserved? Add together the momenta of the objects before the collision and compare this to the momenta of the objects after the collision. Because the red ball is stationary, the total momentum before the collision is that of the green ball: p = (1 kg)×(3 m/s) = 3 kg m/s. After the collision the green ball is stationary, so the total momentum is now simply that of the red ball: p = (1 kg)×(3 m/s) = 3 kg m/s. We can see that momentum is conserved, because it did not change as a result of the collision.
 |
In the illustration above, the collision that is depicted is an elastic collision. As we will learn on page 321, there are three basic categories of collisions: elastic, inelastic, and perfectly inelastic. It is easy to calculate that kinetic energy is conserved in the collision illustrated above, which means that it is an elastic collision. 
|
Whenever analyzing a collision, calculate the momentum for all the objects before the collision and equate that to the momentum of all the objects after the collision. If one object has an unknown momentum, then you can determine it by comparing the total momentum before the collision to the total momentum after the collision.
|
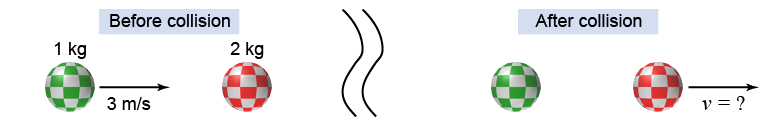In the collision example above, the red ball now has a mass of 2 kg. Once again, the red ball is stationary before the collision and the green ball is stationary after the collision. What will be the velocity of the red ball after the collision? - 1.0 m/s
- 1.5 m/s
- 2.0 m/s
- 3.5 m/s
 |
The correct answer is b. The total momentum before the collision is Set the velocity of the red ball after the collision to v. The total momentum after the collision is Use conservation of momentum to equate the total momentum before and after the collision: Solve for the velocity v of the red ball after the collision: 
|
| |
|
