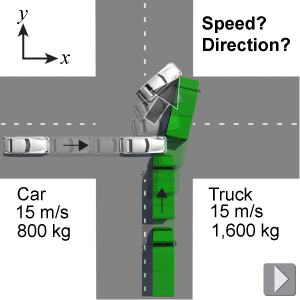|
A passenger car and a pickup truck have collided. Their bumpers lock and the pair skid together across the pavement. Police, insurance agents, and lawyers will analyze the scene in hopes of determining who was at fault. They can determine the post-collision speed from the distance that the two vehicles slid. They also use the direction the vehicles moved after the collision to determine the relative momentum each vehicle had before the accident. The law of conservation of momentum tells the reconstruction team the relationship between the vehicles’ initial speeds and the distance the vehicles traveled after colliding. 
|
|
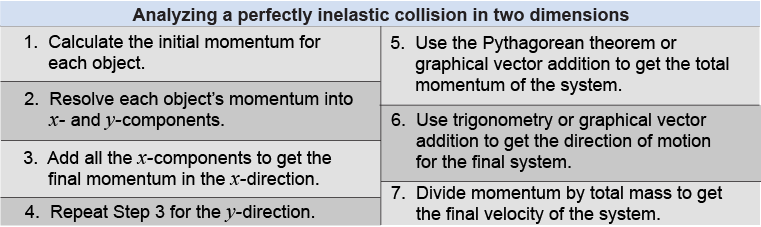
To determine the relative speeds of the vehicles before the collision, the team uses momentum conservation. Since momentum is a vector quantity, the total momentum along each axis must be considered separately.
|
|
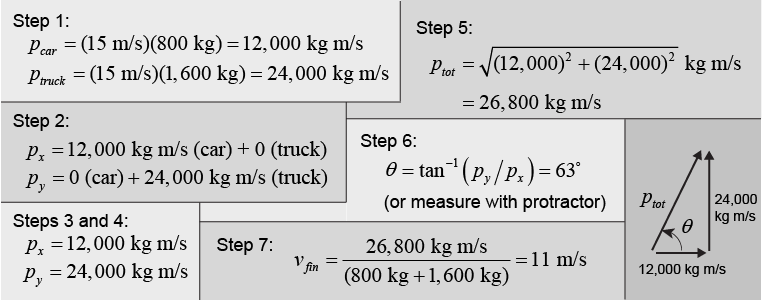
Let’s follow the procedure above for the car–truck collision. Multiply each vehicle’s mass and velocity to calculate its momentum. The car’s momentum is entirely along the x-axis, while the truck’s momentum is along the y-axis. We use the Pythagorean theorem to calculate the total momentum. Since the total momentum is conserved, we equate the total momentum before the collision to the final momentum of the vehicles afterward. We divide the final momentum by the total mass to calculate their mutual velocity after colliding.
 |
As a check, you can compute the initial and final kinetic energies of the system. The total kinetic energy after the collision must not exceed the initial kinetic energy. Indeed, the initial and final kinetic energies of the system can only be equal if the collision is elastic. If the vehicles are deformed or stick together, some of the initial kinetic energy has done work on the vehicle bodies. When that is the case, the final kinetic energy of the system must be less than the initial value. Warning: A common error is to add the vehicles’ velocities, not their momenta. Make sure you calculate each vehicle’s momentum before adding the vectors. 
|
|
Two identical passenger cars enter an intersection at right angles and collide. Car A enters from the west (left), and Car B enters from the south (below). They stick together and skid to the northeast, at a 45° angle to the street grid. Which statement describes their relative speeds prior to the collision? - Car A was traveling faster than Car B.
- The cars were traveling equally fast.
- Car B was traveling faster than Car A.
 |
The correct answer is ii: The two cars were traveling equally fast prior to the collision. Because they slid away from the collision at a 45° angle, they had equal amounts of momentum beforehand; and since they have identical masses, they must have had equal speeds. 
|
A 800 kg passenger car enters an intersection from the west (left) and collides with a northbound pickup truck (whose mass is twice that of the car). The vehicles stick together and skid to the northeast, at a 45° angle to the street grid. Which statement correctly describes their relative speeds prior to the collision? - The car was traveling faster than the truck.
- The car and truck were traveling equally fast.
- The car was traveling more slowly than the truck.
|
|
The correct answer is i: The car had a greater initial speed than the truck. The final velocity vector was at a 45° angle to each vehicle’s initial motion. That means that the vehicles had equal amounts of momentum. But the truck’s mass exceeds that of the car. Since momentum equals mass times speed, the truck’s larger mass must correspond to a slower speed.
|
