|
The momenta of things around us often change. As a car approaches an intersection and slows down, its momentum decreases. A train departs from the station and its momentum increases as its accelerates. A soccer player’s velocity may change direction as she weaves between defenders, and because of this her momentum frequently changes direction. 
|
As we learned on the previous page, impulse J is equivalent to a change in momentum: J = Δp = pf − pi. Imagine a car moving down the street at a velocity of 10 m/s. The driver brakes to a stop; the car’s velocity and hence its momentum have changed. The change in momentum, or impulse J, is the final momentum minus the initial momentum. In the illustration at right, the change in momentum is −20,000 kg m/s. In most problems you will encounter, an object will change its velocity but not its mass. In such cases, a change in velocity means a change in momentum—and that means an impulse has been applied to the object.
|
| | | J | = | impulse (kg m/s) | | Δp | = | change in momentum (kg m/s) | | pf | = | final momentum (kg m/s) | | pi | = | initial momentum (kg m/s) |
| Impulse
change in momentum |
|
|
Impulse has the units of newton seconds (N s). But since impulse is equal to the change in momentum, it also has the same units as momentum, or kilogram meters per second (kg m/s), because 1 N s = 1 kg m/s.
|
|
An impulse can be applied in many different ways. A driver can step on the gas pedal to apply a positive impulse, whereas the brake pedal delivers a negative impulse. Even when slowing down, there are many different ways to deliver the same impulse.
|
|
|
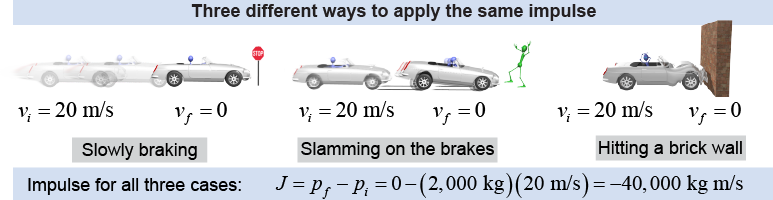
The illustration above shows three examples of how to apply the same impulse to slow down a car. The driver can brake slowly, slam on the brakes, or get into an accident (such as by hitting a brick wall). No matter how his momentum is reduced, the total impulse applied to his car is the same: His momentum changes from 40,000 kg m/s to zero. Even though the impulse applied is the same, the three examples look very different. Why? In each case a different force is applied for a different length of time, but in each case the product of these two quantities equals the change in momentum of the car.
|
A car is traveling east, with positive momentum, when the driver applies the brakes. Is the impulse applied during braking positive or negative?
 |
The impulse imparted on the car is negative. The impulse equation is J = pf – pi. The initial momentum is positive, while the final momentum is zero; therefore, J is negative. 
|
