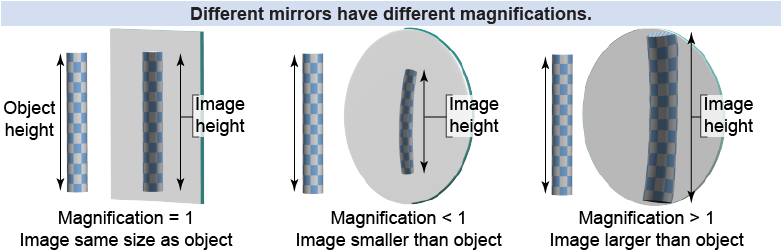
|
If you look in a flat mirror in a dressing room, your image is the same height as you are. A magnifying glass held above this page, however, produces an image of the page that is larger than the page itself. Curved optical elements—lenses and curved mirrors—can be used to create magnified images. In some cases, the magnified image is larger than the object; in others, the image is smaller than the object. 
|
|
|
The magnification of a mirror or lens is the ratio of the image and object heights.
|
| (20.2) | | | m | = | magnification | | hi | = | image size (m) | | ho | = | object size (m) |
| Magnification
|
|
|
Can you hold a concave mirror such that your image is inverted, i.e., upside-down? The values of the heights used in equation (20.2) can be either positive or negative depending on whether the image is upright (positive height) or inverted (negative height). Look at equation (20.2) again: A negative value for hi will produce a negative value for the magnification m. A negative magnification represents an inverted image, while a positive magnification is an upright image.
|
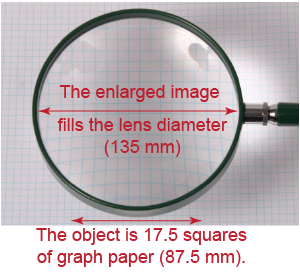What is the magnification of the large magnifying glass in the picture? | Asked: | magnification m | | Given: | Object height: Each graph paper square has sides that are 5 mm long. Since 17.5 squares are visible through the lens, the object “height” is 87.5 mm.
Image height: Since the image fills the lens, the image “height” is 135 mm (27 squares), the diameter of the lens. | | Relationships: | m = hi / ho | | Solution: | | | Answer: | The magnification is 1.54. |
 |
In the solved problem above, it is impossible to tell from the image of graph paper (which is symmetrical!) whether the image created is upright or inverted. As you will learn in the next chapter—or you can discover by experimenting with a real magnifying glass—the biconvex lens in a magnifying glass creates upright images when held close to the paper, but it switches to creating inverted images when held further away. In the solution above, we assumed that the image is upright, which happens to be correct for the particular magnifying glass used in the illustration. 
|
A biologist is observing a plant cell under a microscope. The microscope can be set to 50, 100, or 200 magnification. The cell is 185 micrometers wide (where 1 micrometer = 1 μm = 10−6 m). Which magnification will produce an image of the cell that is closest to 1 centimeter wide?
 |
The biologist should use the magnification of 50.
Asked: which magnification m to use
Given: width of cell, ho = 185 μm = 185×10−6 m; desired image width, hi = 1 cm = 10−2 m;
possible magnifications m = 50, 100, and 200
Relationships: m = hi⁄ho
Solve:
Solve for the ideal magnification: 50 is the closest given magnification to 54.1. 
|
