|
Our interpretation of complex waves as being made of single-frequency components is based on Fourier’s theorem. This theorem says that any repetitive wave can be reproduced exactly by a Fourier series of single-frequency waves with different amplitudes. Fourier’s theorem also includes a mathematical formula for finding the amplitude of each different frequency wave in the series. That is how a frequency spectrum is determined. 
| 
|
|
The top diagram shows the waveform of a 100 Hz square wave that has an amplitude of approximately 120 mPa. The first four sine waves in the Fourier series have frequencies of 100, 300, 500, and 700 Hz and amplitudes of 124, 37, 17, and 9 mPa. These four waves add up to a fairly good approximation of the original square wave. Adding more waves in the series would make the approximation even better.
|
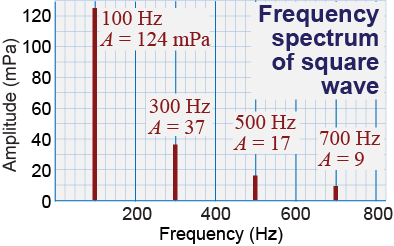The spectrum of a square wave shows the amplitude of each component frequency that makes up the total. The first four largest waves are at frequencies of 100, 300, 500, and 700 Hz. The spectrum is similar to a bar chart, with one vertical bar per frequency. In this chart, the height of the bar represents the amplitude of that frequency. The relative heights of the different frequencies indicate the relationship between the amplitudes of the sound at each frequency. In the spectrum (at right), the fundamental frequency at 100 Hz has the largest amplitude.
|
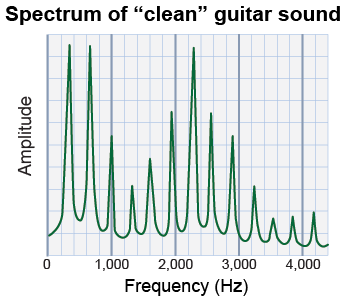Sound ordinarily contains thousands of different frequencies, each with its own amplitude and phase. The spectrum on the right shows the sound wave from an acoustic guitar playing the note E. The frequency spectrum shows that the complex sound of the guitar is made up of many frequencies—similar to the square wave—only with a far more complex mix of different frequencies.
 |
Is there something peculiar about the frequency spectrum of the square wave? The fundamental frequency is 100 Hz while the other harmonics are at frequencies of 300, 500, 700 Hz, etc. What harmonics appear to be missing? The square wave can be produced only with odd harmonics or odd-integer multiples of 100 Hz. It is missing the even harmonics at frequencies of 200, 400, 600 Hz, etc., which are even-integer multiples of 100 Hz. Look closely at the figure above: The guitar’s frequency spectrum includes both odd- and even- integer multiples of the fundamental frequency of approximately 340 Hz. The frequency spectrum of a square wave differs in this basic way from that of the guitar spectrum. If your only data were the frequency spectrum, you could tell a square wave apart from a guitar’s sound wave! 
|
What are the first four frequencies for the Fourier series of a square sound wave with a frequency of 440 Hz?
 |
The first four frequencies are 440, 1,320, 2,200, and 3,080 Hz. The Fourier series is made up of odd multiples of the fundamental frequency. 
|
| |
|
