|
Most structure problems involve at least two equations. At least one equation is force equilibrium, and the other equation is often rotational equilibrium. 
|
As an example, consider a 60 kg person who is standing off-center on a 40 kg scaffold suspended by chains. What is the tension force in each chain?
|
|
The free-body diagram includes two reaction forces from chains, which are treated the same as ropes. The tension forces are not equal because the person is standing off-center. The weight of the scaffold mg is assumed to act at the center, which is 2.5 m from either end. Let the weight of the person be mpg.
|
|
The equation for force equilibrium tells us that the sum of forces in the vertical direction must be zero. (This is true in the horizontal direction, too, but no forces act in either horizontal direction in this problem, so we need not analyze the x-axis.)
|
|
|
The best choice for a center about which to calculate torques is one of the chain attachments. It does not matter which one, so we chose the left support. The sum of torques around this center must be zero when in rotational equilibrium.
|
The torque equation has only one unknown and can therefore be solved for TR. Once TR is known, the force equation is used to solve for TL.
|
|
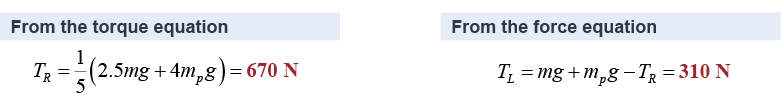
Solving static equilibrium problems starts with determining forces from reactions, weights, and loads. The next step is to apply the equations for equilibrium of forces and equilibrium of torques. You need one equation for each unknown variable. Often, as in this example, one of the equations can be solved directly for one of the variables. The other equations can then be used to solve for the remaining variables.
| |
