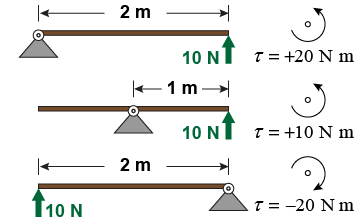|
The same force can produce a different torque around a different center of rotation. The torque from any force depends on the line of action of the force relative to the location of the center of rotation. The drawing to the right shows the same +10 N force creating torques of +20, +10, and −20 N m when applied at three different places with different centers of rotation. 
|
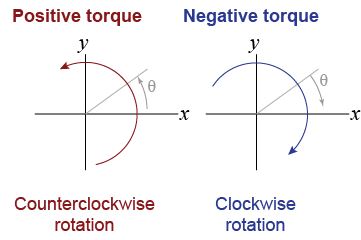Torques can be negative or positive depending on whether they tend to cause clockwise or counterclockwise rotation about any given center. By convention, a positive torque tends to increase the angle with the x-axis, which means counterclockwise rotation. A negative torque tends to decrease the angle with the x-axis, which means clockwise rotation.
|
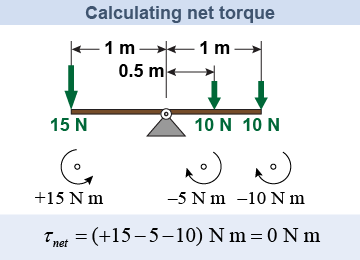Like forces, torques add as vectors. The net torque is the sum of all torques acting around a particular center. Because torque depends on the choice of center, it is absolutely crucial that all torques be determined around the same center point in order to add them. In the example at right of a balancing board, the net torque from the three forces is zero.
 |
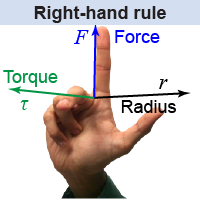If torque is a vector and adds in the same way that vectors add, what is the direction of the torque vector? As you will learn in more advanced physics classes, the direction of the torque vector is determined by the right-hand rule. Use your thumb, index finger, and middle finger as the radius r, applied force F, and resulting torque τ, respectively. If r and F are in the plane of the page, the torque is a vector that goes either into or out of the page, as in the illustrations above and at right. As you can see for these cases, the torque vector is parallel to the axis of rotation. 
|
Torque can be determined around any point in space; it does not have to be the actual center of rotation. You are free to choose the “center” to be anywhere. This is very useful for the following reason:
An object in equilibrium has zero net torque around any center!
If the net torque were not zero around some point in space, the object would rotate around that point. It follows that any object that is not moving must have zero net torque around all possible choices of “center.” For static equilibrium problems it is most convenient to choose the “center” to be the place where one or more unknown forces act. This is useful because the torque is zero for any force whose line of action passes through the center.
|
| |
|
