|
An object is in rotational equilibrium when the net torque on the object is zero about any point (center). In the notation we used for forces, this condition is stated by equation (8.3), which translates to “the sum of the torques is zero.” 
|
| (8.3) | | | Rotational
equilibrium
|
|
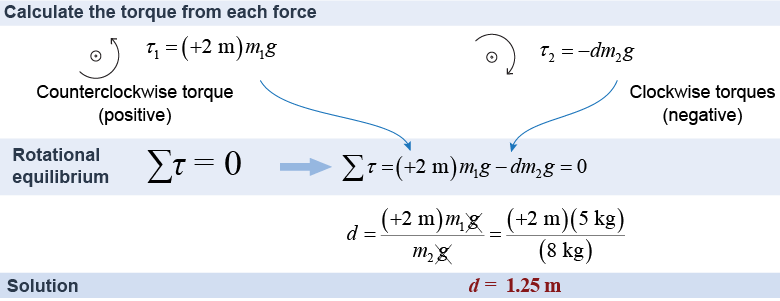
In the problem below, a 5 kg mass is 2 m to the left of the center of a see-saw. Where should the 8 kg mass be placed so the see-saw balances? A see-saw is an example of a lever, which is a board free to rotate around a point called the fulcrum. This is a typical problem of rotational equilibrium. 
|

|
- Draw the free-body diagram and choose a center of rotation.
- Draw each force at approximately the right distance. Label the known and unknown distances.
- Calculate the torque created by each force, being careful to assign positive and negative directions with respect to the center.
- Solve the problem by setting the net torque to zero.

|
|
The 5 kg mass placed 2 m from the fulcrum creates a positive torque of +98 N m. But 8 kg at 1.25 m creates a negative torque of −98 N m. The heavier mass sits closer to the fulcrum than the lighter mass to create equal and opposite torques that cancel out in equilibrium. This is consistent with what you know about see-saws. 
|
If you have a see-saw of length 3 m with a 5 kg weight placed right on top of the fulcrum, where would you put a 4 kg weight to balance it.
 |
You would have to put the 4 kg weight right on top of the 5 kg weight. A weight right on the fulcrum doesn't produce any torque because its distance to the lever arm is zero. This means that the see-saw is already balanced, so putting the new weight anywhere but right on the fulcrum would unbalance it. 
|

