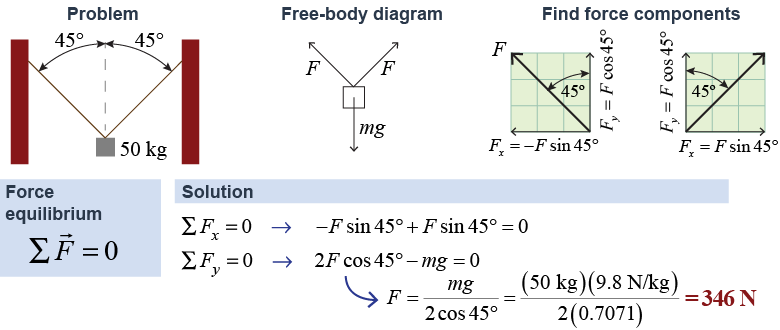
|
To illustrate how to solve equilibrium problems, consider a 50 kg mass hanging from a rope between two walls. What is the tension in each rope in the illustration below, when both ropes make angles of 45° with the vertical? For such equilibrium problems, follow these steps: - Draw the free-body diagram of the mass, replacing the rope with the forces exerted at the proper angles.
- Resolve all forces into x- and y-directions. Use subscripts “x” and “y” to keep track of force components.
- Apply the equilibrium equation to the x- and y-directions to solve the problem.
Once you have a proper free-body diagram, the key step is to resolve all forces into their x- and y-components before setting the net force in each direction to zero. 
|
|
|
In this example, the forces on the left and right ropes are assumed to be equal, and that is why both get the same name, F. This is a good assumption because the problem is symmetric: The left and right forces are reflections of each other. The direction of each force is different but the magnitudes are the same, because both ropes make the same angle with the vertical. Symmetry is often useful in solving equilibrium problems.
|
|
As shown above, the force F is found by applying the equilibrium condition in the y-direction. The vertical (upward) components of the rope tension, 2F cos 45°, balance the vertical (downward) weight of the mass, mg.
|
The problem at right appears at first to be easy to solve using force equilibrium. The pin holding the beam to the wall, however, may exert reaction forces (Rx, Ry) in both the x- and y-directions, so there are three unknown forces—and there is no symmetry we can exploit. A problem with three unknowns needs at least three equations. This problem must be solved using the additional concept of rotational equilibrium.
|
| |
|
