|
How the final position xf depends on the initial position xi and the displacement d can be described by equation (3.1). This equation is a mathematical statement saying “where you end up is where you start plus how much you move.” The distance you move is the absolute value of that displacement. If you changed position from +30 m (initial) to −30 m (final), then the distance you traveled is 60 m but your displacement is −60 m. 
|
| (3.1) | | | xf | = | final position (m) | | xi | = | initial position (m) | | d | = | displacement (m) |
| Position
|
|
In one dimension, displacements add just like positive and negative numbers. The final position is equal to the initial position plus all the successive displacements. For example, suppose an ant starts at 2 m, then moves a displacement of +7.1 m followed by another displacement of −5.5 m. What is its final position? The problem can be solved two ways: graphically and numerically. - To solve numerically, you add the displacements to the initial position, being careful to account for signs. For this example, the final position is 2 + 7.1 − 5.5 = 3.6 m
- To solve graphically, you draw the first displacement to scale starting from the initial position. Each successive displacement is drawn from the end of the previous displacement. The final position is at the end of the last displacement vector.
|
|
|
In many problems either the north–south or the east–west axes are used to define directions. It is common to assign positive values to displacements to the east or north and negative values to displacements to the west or south. This is completely arbitrary! You can choose to define any direction as positive, making the opposite direction negative.
|
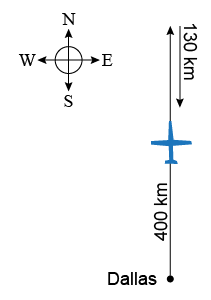An aircraft takes off from Dallas, flies 400 km north, lands, and then takes off again and flies 130 km south. What is the plane’s final position relative to Dallas?
| Asked: | final position xf | | Given: | xi = Dallas, d1 = +400 km, d2 = −130 km | | Relationships: | xf = xi + d | | Solution: | xf = xi + d
→ xf = 0 + 400 km − 130 km = 270 km | | Answer: | The plane is 270 km north of Dallas. |
|
| |
|
