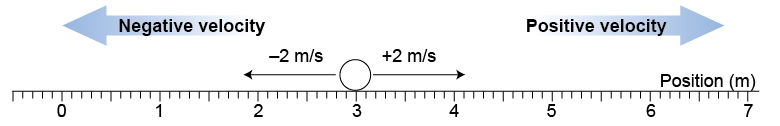
|
Velocity can be positive or negative depending on how you define direction. If moving to the right is defined to be positive, then a negative velocity, such as −2 m/s, describes moving to the left. You should realize that this is a choice and not a rule of physics. You can choose any direction to be positive, including to the left instead of right, or down instead of up. You must be consistent with your choice, however, and not change it in the middle of solving a problem! 
|
|
|
Mathematically, speed is the absolute value of velocity. Velocity has the same units as speed and has the same meaning in terms of describing how fast an object is moving. Velocity has the additional capability of indicating the direction. For one-dimensional motion, the direction of motion is described as positive or negative.
|
Many problems include the term constant speed or constant velocity. This means the value of the velocity v does not change over time. For example, if a problem gives a “constant speed of 10 m/s,” then the speed stays 10 m/s for all times of interest in the problem. Of course, no real speed stays constant for very long; in many circumstances, however, it is a good approximation.
 |
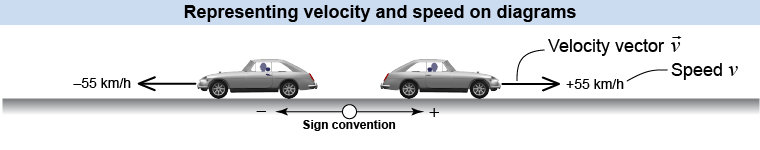The variable for speed is the letter v (for velocity). Velocity is the vector equivalent of speed. Speed is the absolute value or magnitude of velocity. As an example of the difference, the speedometer in a car measures the car’s speed, such as 55 km/hr, but it says nothing about which direction the car is going. To know your velocity you need to also specify the direction, such as 55 km/hr south. In one-dimensional motion the velocity is positive for one direction and negative for the opposite direction. For example, if the velocity for an object traveling north was +55 km/hr then −55 km/hr would mean the same speed but traveling south. With velocities, a sign convention is always implied that defines one direction as positive and the other as negative. In this book, velocity vectors are drawn as black arrows. 
|
|
In many physics problems, it is convenient to set the initial time ti to zero. In such cases, the time interval becomes Δt = t. The initial position (at time zero) may be written as x = x0 or, alternatively, as xi.
|
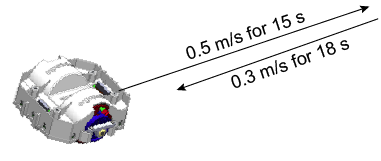A robot travels to the right at a speed of 0.5 m/s for 15 s, then turns around and travels to the left at a speed of 0.3 m/s for 18 s. What is the final position of the robot if it started at x = 0?
|
| Asked: | change in position Δx (or final position since xi = 0) | | Given: | v = 0.5 m/s for 15 s, then v = −0.3 m/s for 18 s. The second speed is expressed as a negative velocity because it is in the opposite direction. | | Relationships: | Δx = v Δt | | Solution: | This problem is solved by calculating two displacements and then adding them. Δx1 = v1Δt1 = (0.5 m/s)(15 s) = 7.5 m
Δx2 = v2Δt2 = (−0.3 m/s)(18 s) = −5.4 m
Δx = Δx1 + Δx2 = 7.5 m − 5.4 m = 2.1 m Since the robot started at position x0 = 0, its final position is: x = 0 + Δx = 2.1 m | | Answer: | The final position of the robot is +2.1 m. |
|
Ryan moves to the right with a positive velocity of 5 m/s for 1 s, then to the left with a negative velocity of −5 m/s for 1 s. What is Ryan’s displacement after 2 s? - 5 m
- 10 m
- 0 m
- −5 m
 |
Answer c is correct, because Ryan’s displacement after 2 s is zero (0 m). 
|
| |
|
