|
Before you can use the information you are given in a physics problem, you must first check the units for consistency. This is very important because not paying attention to units will cause you to fail many physics tests!
To use an equation every quantity must be in consistent units.
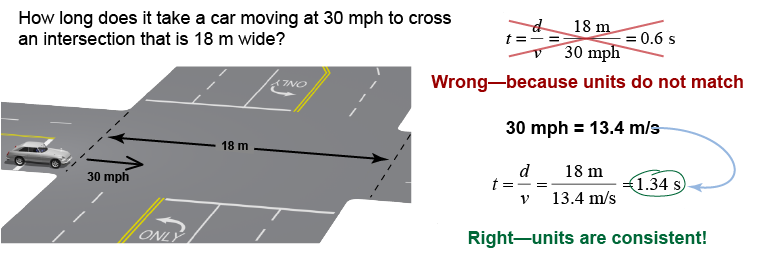
For example, suppose a car is traveling 30 miles per hour. How long does it take the car to cross an intersection that is 18 meters across? Note that speed has units of miles per hour and distance has units of meters. These units are not consistent with each other. If you use the speed formula and calculate the time with speed in miles per hour and distance in meters you will get the wrong answer! To get the correct answer you have two choices. You can convert the speed of the car into meters per second to match the distance across the intersection in meters, or you could convert the distance across the intersection into miles to match the speed in miles per hour. If you use this second choice your time will come out in hours! 
|
Speed has units of distance divided by time. When converting speeds, you need to use conversion factors for both time and distance. To convert the distance, you might use the conversion factor 1 mile = 1,609 meters. For time, you might use 1 hour = 3,600 seconds. The three calculations on the right show correct and incorrect ways to do the conversion. Which is correct?
|
|
The correct conversion is the one in which all the units cancel out—except for meters in the numerator and seconds in the denominator. Once the conversion factors are arranged so that the units work out, all you have to do is multiply and divide the values as they appear in the numerator or denominator. In this example, the speed of the car is 13.4 m/s. Once the speed has been converted to units of meters per second it is consistent with the distance across the intersection in meters. To solve the problem you apply the formula t = d/v to calculate that it takes the car 1.34 s to cross the intersection.
|
In the USA, gas efficiency is measured in miles per gallon. In Europe, it is measured in kilometers per liter. If a car’s gas mileage is 25 mi/gal, then how many liters of gasoline would you use over a 133 km trip? Use 1 km = 0.62 mi and 1 gal = 3.78 L. - 9.59 L
- 13.25 L
- 12.46 L
- 10.67 L
 |
The correct answer is c. This problem has multiple conversions that must be made. To avoid error it is best to do them step by step. First we will convert mi/gal to km/gal: Now we can convert km/gal to km/L: Now with the correct units we can calculate how many liters of gasoline are needed to travel 133 km: 
| |
| |
|
