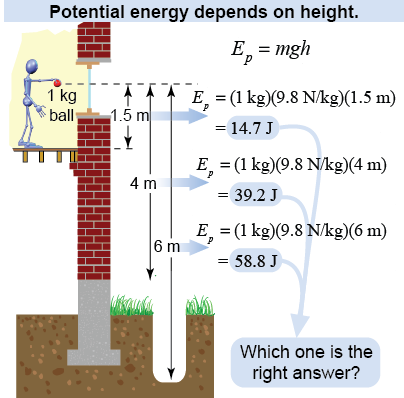|
Hold a ball above the ground. What is the ball’s potential energy before you drop it? It depends! The ball is not at zero height—if it was it couldn’t have fallen because it would have already been on the ground. For that matter, even the ground is not truly zero height because you could dig a hole and let the ball fall farther into the hole. Depending on your choice of coordinate system, the ball’s height—and hence its potential energy—varies. From the perspective of potential energy, where is “zero height”? Is there even such a thing? 
|
|
The answer to that question is that the energy of a system depends on the reference frame in which things are measured. For example, if the reference frame is the room, a 1 kg ball that is 1 m from the floor has a potential energy of 9.8 J. If the reference frame is the ground two stories down, the potential energy is 68.6 J. The actual position of the ball is the same but the choice of reference frames is different.
|
|
The choice of a reference frame (such as where “zero” is) is arbitrary because it is differences in energy that matter, not absolute values. A ball that falls 1 m loses 9.8 J of potential energy. It does not matter if the ball falls from 1 m to zero or from 7 m to 6 m. The amount of potential energy lost or gained from a change in height is independent of where zero is defined. We are therefore free to choose any reference frame that makes the calculation easiest! The only rule is that the reference frame should not change in the middle of a problem.
|
You might think kinetic energy is independent of reference frames. A ball at rest in your hand has zero velocity, doesn’t it? In your reference frame, which is fixed to the Earth, the ball’s kinetic energy is zero. Consider, however, that Earth is moving through space at 29,780 m/s. Relative to the Solar System, the ball on the table has a kinetic energy of 443 × 106 J! Like potential energy, kinetic energy also depends on the reference frame in which speed is measured.
 |
One of the key points of Einstein’s theory of relativity is that any physical observation or experiment has the exact same result regardless of any uniform speed of the reference frame in which the experiment is carried out. Again, because it is energy differences that matter, the choice of what is considered “zero velocity” is as arbitrary as choosing what is zero height. Only in extreme cases, such as speeds near the speed of light or near a black hole, are there differences from the relative motion of reference frames. These differences are completely unnoticeable in ordinary experience. 
|
A 60 kg man is sitting on the floor of an airplane 8,000 m high traveling at 850 km/hr. How much total mechanical energy does he have relative to the reference frame of the plane? - 0 J
- 1,700,000 J
- 4,700,000 J
- 6,400,000 J
 |
Relative to the plane, the man is at height 0 and is traveling with velocity 0, so his total mechanical energy is also 0. 
|
| |
|
