|
Kinetic energy (Ek or KE) is the energy of motion. Any object that has mass and is moving has kinetic energy because it is moving. When you catch a ball, your hand applies a force over some distance (your hand recoils a bit) to stop the ball. That force multiplied by the distance represents the transfer of the ball’s kinetic energy to your hand by doing work on your hand. Now think about catching balls of different masses and speeds. You can probably guess that a massive or a fast-moving ball has more kinetic energy—it is harder to stop!—than a lighter, slower ball. 
|
| (9.2) | | | Ek | = | kinetic energy (J) | | m | = | mass (kg) | | v | = | speed (m/s) |
| Kinetic energy
|
|
|
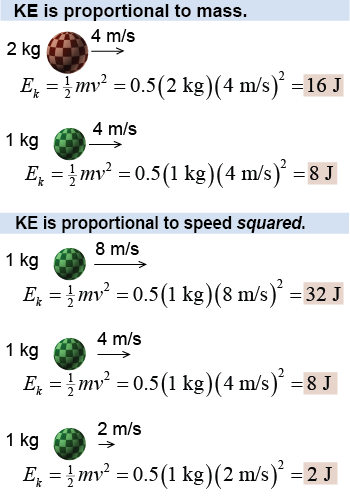
Kinetic energy is calculated using equation (9.2) above. The kinetic energy of a moving object is proportional to mass. If you double the mass, you double the kinetic energy. For example, a 2 kg ball moving at a speed of 1 m/s has 1 J of kinetic energy according to the equation. A 4 kg ball moving at the same speed has 2 J of kinetic energy, or twice as much. This is a linear relationship.
| 
|
According to equation (9.2) kinetic energy depends on the square of the speed of a moving object. Consider a 2 kg ball traveling at 1 m/s with 1 J of kinetic energy. The same ball moving at 3 m/s has 9 J of kinetic energy. If you multiply the speed by 3, then the kinetic energy is multiplied by a factor of 32 = 9. This is an example of a nonlinear relationship.
 |
A linear relationship—i.e., a straight line on an x–y graph—can be represented by the formula y = mx + b. If you change x by a factor of 2, then y changes by a factor of 2m; if you change x by a factor of 3, then y changes by a factor of 3m; and so on. In this linear relationship, y is a function of x—where x is raised to the first power.
In a nonlinear relationship, however, y is a function of x raised to some other power—such as x2, or x7, or . Whereas the x–y graph of a linear relationship such as y = x is a straight line, the x–y graph of nonlinear relationship such as y = x2 is a curved line (a parabola in this case). The equation for kinetic energy shows that kinetic energy is a nonlinear function of velocity v (because it depends on velocity squared v2), but it is a linear function of mass m. 
|
|
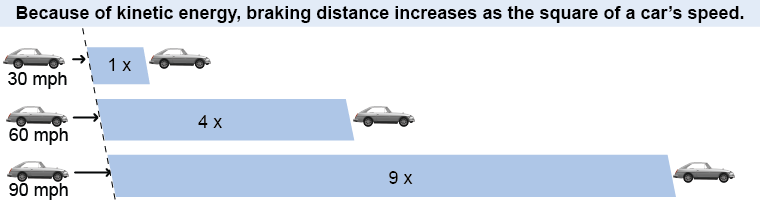
The fact that kinetic energy increases with the square of the speed has implications for the stopping distance of a car. As a car brakes, work is done to transform the car’s kinetic energy into thermal energy. Work is force multiplied by distance. Assuming that the braking and road conditions result in a relatively constant stopping force, then the distance it takes the car to stop is proportional to the initial kinetic energy. At 30 mph a car can stop in about 15 m. When the speed is doubled to 60 mph (and the kinetic energy increases by a factor of 4) it takes four times as much distance to stop.
|
|
A motorcycle has 10 J of kinetic energy. If its velocity is doubled, how much kinetic energy does it now possess? - 15 J
- 20 J
- 35 J
- 40 J
 |
The answer is d, 40 J. Because velocity is a squared term in the kinetic energy formula, when it is doubled, kinetic energy increases by a multiple of 4. 
|
