Essential Physics

Section 3 review


Solving physics problems usually involves translating the language of the problem into mathematical statements: identify what is asked for in the question; determine what quantities and other information are given to you; identify key relationships among the quantities; and solve the problem. In the process, it is important to assign variables to quantities, make clear and correct assumptions, and use consistent units.
Review problems and questions

A student is biking to school. She travels 0.7 km north, then realizes something has fallen out of her bag. She travels 0.3 km south to retrieve her item. She then travels 0.4 mi north to arrive at school. The journey takes her 15 min. What is her total displacement? What is her average velocity?
Answer: 1.0 km 4 km/hr Solution: Define north as positive and south as negative. Asked: Total displacement \( \vec{d_t} \) student travels Given: Displacement at three different times, \( \vec{d_1} \) = 0.7 km, \( \vec{d_2} \) = −0.3 km, \( \vec{d_3} \) = 0.4 mi Relationships: \( \vec{d_t} \) = \( \vec{d_1} \) + \( \vec{d_2} \) + \( \vec{d_3} \) , 1 mi = 1.6 km Solve: \( \vec{d_3} \) = 0.4 mi = (0.4)(1.6 km) = 0.6 km \( \vec{d_t} \) = 0.7 km − 0.3 km + 0.6 km = 1.0 km Answer: \( \vec{d_t} \) = 1.0 km Use answers from above. Asked: Average velocity v student travels Given: Overall time t = 15 min, total displacement \( \vec{d_t} \) = 1.0 km Relationships: v = \( \vec{d_t} \) /t, 1 hr = 60 min Solve: t = 15 min = (15/60) hr = 0.25 hr; v = (1.0 km) / (0.25 hr) = 4 km/hr Answer: v = 4 km/hr Note that the total distance (not displacement) she traveled was 0.7 + 0.3 + 0.6 = 1.6 km and that the average speed (not velocity) that she traveled was (1.6 km) / (0.25 hr) = 6.4 km/hr.
Two students travel from school to their jobs at the bowling alley, which is 2.5 km away. One student rides a scooter at 9.0 m/s, and the other student rides a bike at 12 mi/hr. Who arrives at the bowling alley first? How long does the trip take for each of them?
Answer: The student on the scooter arrives first. The trip takes 4.6 min (278 s) for the student on the scooter and 7.8 min (466 s) for the student riding a bike.
Two cars are initially separated by 1.0 km and traveling toward each other, one at 30 mi/hr and the other at 30 km/hr. How far in kilometers from their respective starting points do the two cars meet?
Answer: When they meet, car₁ is 0.63 km from its starting point and car₂ is 0.39 km from its starting point. Solution: Asked: Distance d_f1 and d_f2 from starting points car₁ and car₂ will be when they meet Given: Car₁’s speed v₁ = 30 mi/hr, car₂’s speed v₂ = 30 km/hr, start distance d_i = 1 m from each other Relationships: v = d/t, 1 mi = 1.61 km Solve: $v = \frac{d}{t}$ Solve for t: $t = \frac{d}{v}$ Before proceeding we must make certain that all units are consistent. So we must convert v₁ to km/hr. $v_{1} = (30 mi/hr) (\frac{1.61 km}{1 mi}) = 48.3 km/hr$ How long does it take the cars to meet: $t = \frac{d_{i}}{v_{1} + v_{2}} = \frac{1 km}{48.3 km/hr + 30 km/hr} = 0.013 hr$ Solve for the distance each car is from its starting point with v = d/t, solve for d: $d = v t$ Solve for d_f1 of car₁: $d_{f 1} = v_{1} t = 48.3 \frac{km}{hr} \times 0 .013 hr =0 .6279 km$ Solve for d_f2 of car₂: $d_{f 2} = v_{2} t = 30 km/hr \times 0.013 hr = 0.39 km$ Answer: When they meet, car₁ is 0.63 km from its starting point and car₂ is 0.39 km from its starting point. Note: The two distances do not add up to one because of rounding errors between steps and with the mile-to-kilometer conversion factor.
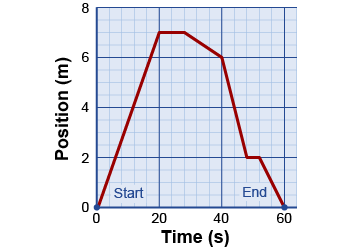
A student was maneuvering the ErgoBot and generated the position versus time chart illustrated at right. Describe in words the motion of the ErgoBot for each segment of the chart.
The ErgoBot started moving forward, moving 7 m in 20 s at a steady speed of 0.35 m/s. It then stopped for 8 s. It then moved slowly 1 m backward at a speed of 0.083 m/s for 12 s. It then moved backward 4 m at a speed of 0.5 m/s over a period of 8 s. Then the ErgoBot stopped for 4 s. Finally, the ErgoBot moved backward 2 m for 8 s at a speed of 0.25 m/s.
An airplane flew at a speed of 500 km/hr for 1.5 hr. It then hit a tail wind that boosted its speed to 650 km/hr for the next 750 km. How far did it travel during the first stage of its trip? How long did it take to complete the second stage (only) of its trip? How far did it travel during the entire trip? How long did it take for the entire trip? What was its average speed for the trip?
Answer: 750 km 1.15 hr 1,500 km 2.65 hr 566 km/hr Solution: Distance is speed multiplied by time: $d = v t = (500 km/hr)(1 .5 hr) = 750 km$ Divide both sides of the equation by v to solve for t: $\begin{matrix} d \times \frac{1}{v} = v t \times \frac{1}{v} & \Rightarrow & t = \frac{d}{v} = \frac{750 km}{650 km/hr} = 1.15 hr \end{matrix}$ The total distance traveled is 750 km + 750 km = 1,500 km. The total time traveled is 1.5 hr + 1.15 hr = 2.65 hr. The average velocity is therefore: $v = \frac{d}{t} = \frac{1, 500 km}{2.65 hr} = 566 km/hr$
Take a Quiz


		95