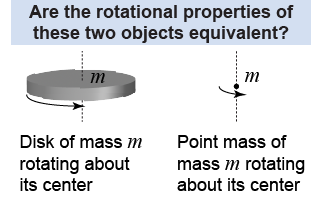Moment of inertia of common objects
Point masses and rotation Are the rotational properties of these two objects equivalent? In Newtonian mechanics you can often simplify a situation by replacing an object with a point mass located at its center of gravity. Now think about the rotation of a disk about its center axis: Can we replace the disk with a point mass at its center? No! The disk possesses a moment of inertia about the axis that depends on how the mass of the disk is distributed in space. In rigid-body rotation, we cannot replace objects with point masses because the shape of the object is important. Read the text aloud
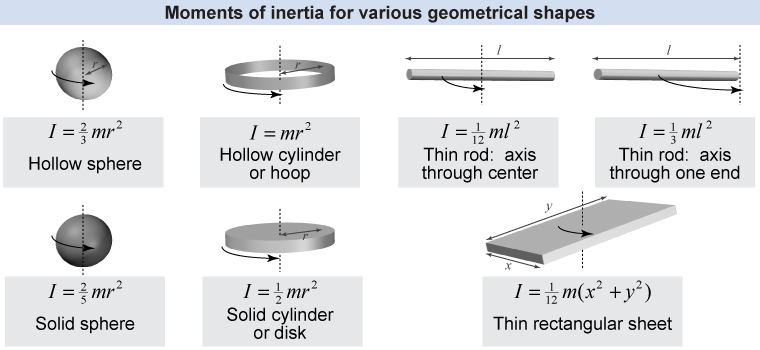
Moment of inertia for distributions of mass Every object has a center of mass; likewise, every object has a moment of inertia when it is rotated about a particular axis. The illustration below shows the moment of inertia for several geometrical shapes. Look at the two cases of the rotation of a rigid rod: The moment of inertia is different depending on which axis you use! The moment of inertia is an important quantity to understand when designing a device that rotates! Read the text aloud
Moment of inertia for various geometrical shapes about different axes of rotation
Angular momentum and moment of inertia The moment of inertia is a convenient quantity for describing the rotational motion of rigid bodies. As we saw on the previous page, angular momentum can also be expressed as the product of moment of inertia and angular velocity, or L = Iω. Read the text aloud
Comparing moments of inertia A hollow and a solid sphere, each with a radius of 10 cm and mass of 0.3 kg, are rotating at 2 rad/s about an axis passing through their centers. Which has more angular momentum?
Asked: angular momenta of a hollow sphere, Lh, and a solid sphere, Ls
Given: radius r = 0.1 m, mass m = 0.3 kg, angular velocity ω = 2 rad/s
Relationships: angular momentum L = Iω; moments of inertia of hollow sphere,
I h = 2 3 m r 2 , and solid sphere, I s = 2 5 m r 2
Solution: L h = I h ω= 2 3 m r 2 ω= 2 3 (0.3 kg) ( 0.1 m ) 2 (2 rad/s)=0.004  kg m 2 /s L s = I s ω= 2 5 m r 2 ω= 2 5 (0.3 kg) ( 0.1 m ) 2 (2 rad/s)=0.0024  kg m 2 /s
Answer: The hollow sphere has a larger angular momentum.
Read the text aloud
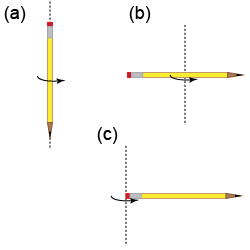Test your knowledge Three different rotational axes for a rotating pencil Consider rotating a pencil about
  1. an axis passing lengthwise through its center,
  2. an axis passing through its center but perpendicular to its length,
  3. an axis through one end and perpendicular to its length.
What is the order of increasing moment of inertia for a–c? Show
Previous Page Next Page375