|
Did you walk up a simple machine today? This might sound like a strange question, but in all likelihood you did. An inclined plane—or ramp—is a type of simple machine. If you walked up a wheelchair access ramp, or even walked on a road up a hill, your path was a simple machine! Ramps are machines because less force is needed to move up a ramp than is needed to climb directly up the same vertical distance. Ramps are used to move cars onto auto carriers, move dump trucks out of mining pits, or move vehicles on roads over mountain passes. 
|
Ramps as simple machines
|
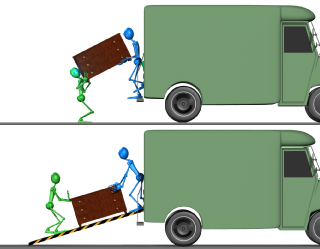Consider two ways to lift a heavy dresser onto a truck. Lifting it vertically requires a force equal to the weight of the dresser. A better idea is to use a ramp. A ramp uses an angled surface to divide the weight force into two directions: parallel and perpendicular to the ramp. The perpendicular force is supported by the normal force from the ramp. The dresser can now be moved along the ramp by overcoming only friction and the (much smaller) parallel force.
|
|
It takes a little experimentation to discover that the steeper the slope of the ramp the more force is required to move up it. How can this intuition be converted into an equation for mechanical advantage of the ramp? The input work done by using the ramp is force times distance, or FiL, where L is the length of the ramp. The output work represents what would happen if you had no machine to help you, or Foh, where h is the vertical height it is being lifted. The gravitational potential energy gained by the dresser will be the same mgh whether you use a ramp or lift it directly into the truck. Since changes in energy are equivalent to work done, both ways of doing work are equal, or FiL = Foh. Mechanical advantage is the ratio of output force to input force, so rearranging terms leads to equation (12.7).
|
| (12.7) | | | MAramp | = | mechanical advantage | | Lramp | = | length of ramp (m) | | hramp | = | height of ramp (m) |
| Mechanical advantage
of a ramp |
|
A road through the Rocky Mountains goes up the side of a mountain pass using switchbacks. A road sign says that it is a 5% grade; i.e., there is a 5 m gain in altitude for every 100 m of distance along the road. What is the mechanical advantage of the road?
 |
Answer: The mechanical advantage of the road is 20.
Asked: mechanical advantage MA of the road
Given: grade of the road, G = 5% = 0.05
Relationships: mechanical advantage of an inclined plane, MA = L/h
Solution: The grade of the road tells you that for every L = 100 m of distance along the roadway there is an increase in height of h = 5 m. The roadway is a ramp, which has a mechanical advantage of MA = L/h = (100 m)/(5 m) = 20. 
|
