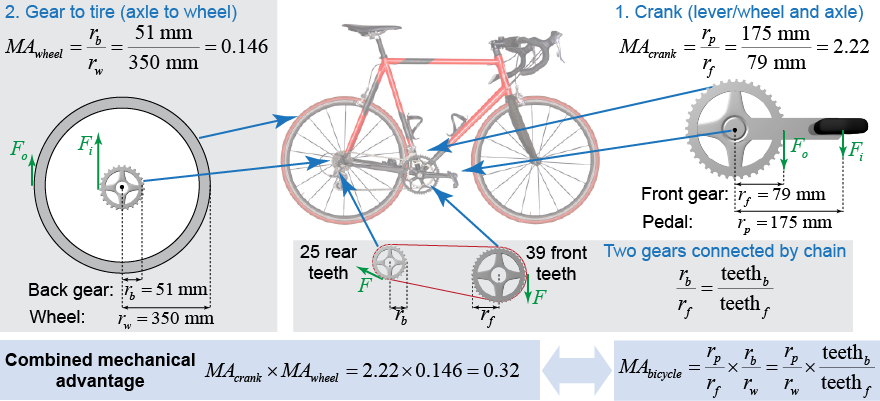
|
|
When you pedal a bicycle, you cause the rear wheel to turn. How is the motion of your feet converted into forward motion of the bicycle? The moving parts of the bicycle that connect the pedals to the wheel are called the drive train. 
|
|
The crank is a lever integrated into a wheel-and-axle simple machine. Pushing down the pedal provides the input force and the output force turns the front gear. The mechanical advantage of the bicycle crank is the ratio of the radius of the pedal to the radius of the front gear, or MAcrank = rp / rf.
|
|
The back wheel is another wheel-and-axle simple machine, but this time the input force turns the back gear (the axle) and the output force is exerted by the tire on the outside of the wheel. The mechanical advantage of the back wheel is the ratio of the radius of the back gear to the radius of the wheel, or MAwheel = rb / rw.
|
The chain connects the front gear to the back gear such that the force is the same everywhere in the chain. Thus the combined mechanical advantage of a bicycle is the product of the mechanical advantages of the crank and the back wheel: | |
Since the teeth are the same size on the front and back gears—the same chain runs over both gears!—the ratio of the radii of the back gear to the front gear, rb / rf , is the same as the ratio of their number of teeth. We can substitute the number of teeth for the ratio of the gear radii to get the mechanical advantage of a bicycle:
|
| | Mechanical advantage
of a bicycle |
|
The mechanical advantage of a bicycle depends on the ratio of the number of teeth in the back and front gears. Now we can see why most modern road bicycles have more than one gear! When you change gears on a bicycle, you are changing its mechanical advantage.
 |
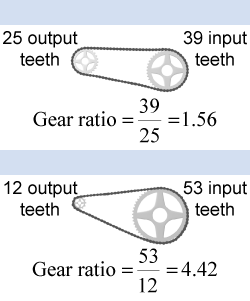Modern road bicycles have two or three different front gears (on the crank) called the chainrings, as well as seven to ten gears on the back wheel called the cassette. By changing the front gear, the back gear, or both, a cyclist can change the mechanical advantage of the bicycle. A common configuration for a road bike has chainrings of 39 and 53 teeth and a cassette with gears ranging from 12 to 25 teeth. These gears can create gear ratios that vary by nearly a factor of 3, from 1.56 to 4.42. Likewise, the mechanical advantage of the bicycle varies by nearly a factor of 3. 
|
- Calculate the mechanical advantage for the road bike in the illustration at the top of the page if the front gear were to be on the large chainring (53 teeth) while the back gear were to be on the smallest gear of the cassette (12 teeth).
- Compare this mechanical advantage to the example in the illustration at the top of the page, which used the small chainring for the front gear (39 teeth) and the largest gear on the back cassette (25 teeth). Which has a larger mechanical advantage?
- In what situations would you want to use each of these gear combinations? Why?
 |
- Using the equation for mechanical advantage gives So the mechanical advantage is 0.113.
- The mechanical advantage of the example in the illustration is larger than the mechanical advantage in Part a.
- Larger mechanical advantages require less force, but they require that the input moves a further distance. Mechanical advantage is a tradeoff of force for distance—or force for speed, since speed is distance over time. You use the small front gear and large rear gear when you are moving slowly and want to apply less force, such as when climbing a steep hill. You use the large front gear and small rear gear when you are moving fast and can apply more force, such as when you are going downhill.
|
