|
A gear is a wheel with teeth that interlock with matching teeth on a mating gear to transmit rotating motion and torque. Gears can transmit much higher forces than smooth wheels. Gears are used to change rotational forces and speeds in many machines, including automobile transmissions. Related to the gear is the sprocket, which is found in the drive train of a bicycle. A sprocket is a toothed wheel that engages a chain instead of another gear. 
|
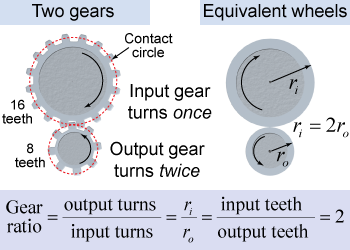Interlocking gears with different numbers of teeth turn at different rotational speeds. The gear ratio is the ratio of turns of the output gear to turns of the input gear. The gear ratio in the example is 16 ÷ 8 = 2. Each turn of the input gear moves 16 teeth through the point of contact, forcing the output gear to turn twice. The gear ratio is the inverse of the ratio of teeth. Note that, like two touching wheels, interlocking gears always rotate in opposite directions.
|
| | | Gear ratio
|
|
The input gear’s teeth apply a force to the output gear’s teeth at the point of contact. At this point of contact, the input force equals the output force (if 100% efficiency is assumed). If the radii of the input and output gears differ, then the input and output torques will differ, too, because torque is force times radius. The mechanical advantage of two gears is the ratio of the output to input torque, which is equal to MA = ro/ri or 1/GR. As you can see in the illustration at right, the MA is also the ratio of the number of output teeth to the number of input teeth.
|
| (12.6) | | | MAg | = | mechanical advantage | | τo | = | output torque (N m) | | τi | = | input torque (N m) |
| MA of
two gears |
|
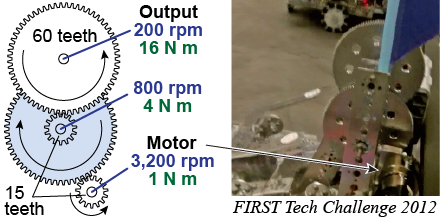Small electric motors turn at high speeds but produce low torque. Many robotic applications require low speed and high torque. Multiple gear ratios are used to both reduce the speed and increase the torque. The four gears in the diagram make an overall mechanical advantage of 16.
 |
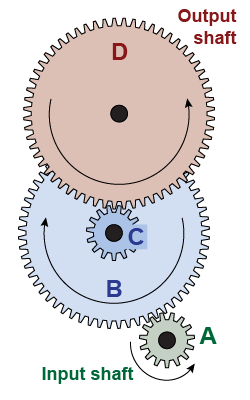The key to understanding this compound gear ratio is to recognize that the two blue gears are fixed together and so turn at the same rate. Suppose gear A turns 16 times. Gear B has four times as many teeth so it turns only 4 times. Gear C is fixed to gear B and also turns four times. Gear C engages gear D, which then turns once since gear D and gear C make a ratio of 4/1. The overall gear ratio between the input shaft (gear A) and the output shaft (gear D) is 4 × 4 = 16.
It is generally impractical to design a large gear ratio, such as 16:1, with only two gears. Consider that it is impossible to make a functional gear with one tooth, or even four teeth. (Think about it!) Assume that the smallest practical gear has 10 teeth. A ratio of 16:1 requires a second gear with 160 teeth. A 160-tooth gear is both large and expensive. The same mechanical advantage can be achieved with two 10-tooth gears and two 40-tooth gears arranged in two ratios similar to those in the diagram. The four-gear design is much smaller and considerably less expensive to make. 
|
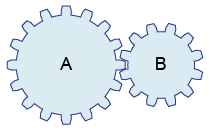For the two gears at right, what is the - gear ratio if A is the input gear?
- mechanical advantage if A is the input gear?
- gear ratio if B is the input gear?
- mechanical advantage if B is the input gear?
 |
From inspection of the figure, A has 16 teeth and B has 12 teeth. - The gear ratio is the ratio of input teeth to output teeth, or 16/12 = 4/3 when A drives B.
- The mechanical advantage is the ratio of output teeth to input teeth, or 12/16 = 3/4 when A drives B.
- The gear ratio is the ratio of input teeth to output teeth, or 12/16 = 3/4 when B drives A.
- The mechanical advantage is the ratio of output teeth to input teeth, or 16/12 = 4/3 when B drives A.
|
