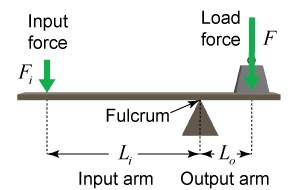|
A lever is a rigid beam that can rotate around a point called the fulcrum. In the example on the right, the input force on one side is in equilibrium with the load force on the other side. The input arm is the distance between the fulcrum and the input force, while the output arm is the distance between the fulcrum and the load force. 
|
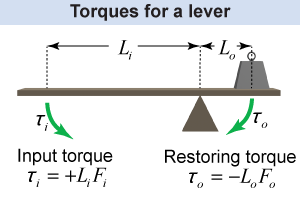The key to understanding how a lever works is that the input torque on one side must balance with the torque from the load force on the other side. As we learned in Chapter 8, torque is the product of force applied and distance from the fulcrum. The input force produces a torque τi = +LiFi while the torque from the load is τo = −LoFo. To be in rotational equilibrium, the net torque must be zero:
or LiFi = LoFo. Rearranging terms leads to the mechanical advantage of a lever in equation (12.2).
|
| (12.2) | | | MAlever | = | mechanical advantage | | Li | = | input arm (m) | | Lo | = | output arm (m) |
| Mechanical advantage
of a lever |
|
|
The great fact about levers is that the mechanical advantage depends only on the relative lengths of the input and output arms—in other words, the lever’s mechanical advantage depends on where you place the fulcrum! If the fulcrum is placed close to the output force, then Lo > Li and MA > 1. To increase the mechanical advantage of a lever, it is only necessary to move the fulcrum of the lever even closer to the output force (the “load”).
|
Where should Michellin place the fulcrum when using a meter stick to construct a lever with MA = 4?
| Asked: | location of the lever’s fulcrum, i.e., the length of the output arm, Lo | | Given: | total length of lever, L = 1 m; mechanical advantage MAlever = 4 | | Relationships: | MAlever = Li/Lo; and length of meter rule, L = Li + Lo | | Solution: | Solve for the length of the input arm: Li = MAlever×Lo = 4Lo Use this to substitute for Li: | | Answer: | The fulcrum should be placed 0.2 m from the output end of the meter stick. |
|
During an investigation, Bobbi placed the input mass at the 90 cm mark of a meter stick, the output mass at the 20 cm mark, and the fulcrum at the 65 cm mark. - What is the length of the input arm of her lever?
- What is the length of the output arm of her lever?
- What is the mechanical advantage of the lever?
 |
- The input arm is the distance between the input mass and the fulcrum, or 90 cm − 65 cm = 25 cm.
- The output arm is the distance between the output mass and the fulcrum, or 65 cm − 20 cm = 45 cm.
- The mechanical advantage is the ratio of the input arm to the output arm, or (25 cm)/(45 cm) = 0.56.
|
