|
Rolling friction occurs when two surfaces are in rolling contact with each other. Wheels are found throughout human technology because rolling motion usually results in much lower friction than sliding. Rolling friction is more complex than static or kinetic friction because the radius of the wheel matters as well as the nature of the contacting surfaces. This is because rolling friction comes from deformation and larger wheels deform proportionally less than smaller wheels. The force of rolling friction is approximately given by equation (5.7). 
|
| (5.7) | | | Ff | = | force of rolling friction (N) | | μr | = | coefficient of rolling resistance | | FN | = | normal force (N) |
| Rolling friction
|
|
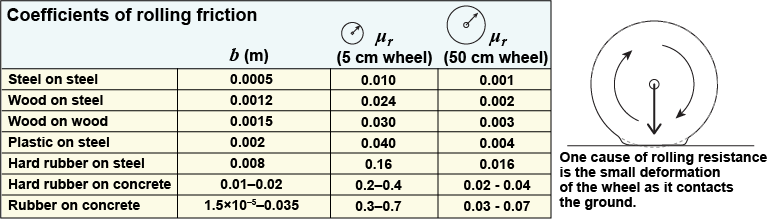
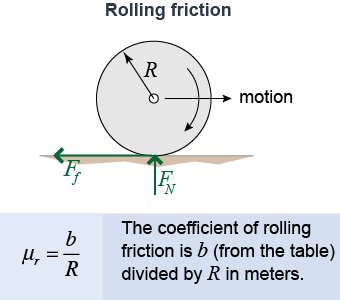The coefficient of rolling friction μr depends on the size of the wheel. The parameter b is used to calculate a value for μr if you know the radius of the wheel in meters. The coefficient of rolling friction is μr = b/R, where b and R are both measured in meters. This causes the units of b and R to cancel to make μr dimensionless. The table below gives some values for wheels of 5 and 50 cm radius. You can see that the coefficient of rolling friction drops by a factor of 10 when the wheel radius increases from 5 to 50 cm.
|
|
A bicycle has two wheels 74 cm in diameter. What is the force of rolling friction on a level road if the weight of the bicycle and rider is 700 N? | Asked: | friction force Ff | | Given: | R = 0.74 m, FN = 700 N | | Relationships: | Ff = μrFN, μr = b/R | | Solution: | Assuming rubber on concrete, μr = b/R = (0.035) ÷ (0.74) = 0.047, so Ff = μrFN = (0.047)(700 N) = 33 N |
|
The models for static, kinetic, and rolling friction are useful for making estimates. These models are not rigorously obeyed natural laws such as F = ma. Friction models are at best rough approximations of the actual friction in real-world situations. Friction is very dependent on the precise conditions of the surfaces, their temperature, and the presence of oils and dirt.
 |
In all three friction models we made assumptions that are not always valid. One assumption is that both static and kinetic friction are independent of the area that actually touches between the two surfaces. This is approximately true only for relatively small, totally rigid, smooth surfaces. It is not true at all for soft surfaces that can conform, such as rubber. In the kinetic and rolling friction models, we also assumed that friction is independent of speed. Some types of friction increase when the motion becomes faster—including air friction and fluid friction. Other types of friction decrease with speed. In any real situation you would measure the coefficient of friction with the exact surfaces in question under a range of conditions. 
|
