|
The largest inefficiency in transportation vehicles, such as cars, trucks, and planes, involves overcoming air resistance. In boats, friction forces are even larger because water is both denser and more viscous than air. Friction from fluids, such as water or air, is caused by two factors: - the shear resistance of the fluid from viscosity and
- the effort required to push the fluid out of the way.
|
The viscosity of a fluid describes its resistance to flow. “Thick” fluids such as honey have a high viscosity and flow very slowly. “Thin” fluids such as water have a low viscosity and flow much faster under the same conditions. Viscosity depends strongly on temperature and is a major factor in lubricating oils for engines. The SI unit for viscosity is pascal-second (Pa s), but a commonly-used unit is the poise, which is equal to 0.1 Pa s. The specification “10W30” in a motor oil means the viscosity of the oil at 0ºC (“W” means “winter”) is no more than 3.1 poise while the viscosity at 100ºC is no less than 0.1 poise.
 |
Motor oils have a standard grading system for how their viscosity varies with temperature. A #10 oil has a viscosity of 3.1 poise at 0ºC and a viscosity of 0.05 poise at 100ºC. This is too thin for most engines to provide proper lubrication for bearings. A “heavier” #30 oil has the right viscosity when warm (0.1 poise) but is too thick when cold (15.3 poise) to be pumped through the engine. In the early 1950’s several companies invented “multigrade” oils that are thinner when cold and thicker when warm than the standard weights of single-grade oils that existed at the time. Multigrade oils include special chemical compounds that increase the viscosity when the oil is hot, allowing a thinner oil to perform adequately at higher temperatures. 
|
For vehicles moving through water or air, the force of air resistance is dominated by inertial effects—essentially the force needed to push the fluid out of the way. The amount and rate at which air or water must be pushed out of the way depend on the shape and size of the object and also on the velocity of the object. When an object moves faster through a fluid, two factors contribute to increasing the air resistance: - more fluid must be displaced per second and
- fluid must be accelerated out of the way more rapidly.
|
|
For a given shape, fluid friction increases as the square of the speed. Doubling the speed of a car from 30 to 60 mph increases the air resistance by a factor of 4.
|
| (5.8) | | | Ff | = | friction force (N) | | cd | = | drag coefficient | | ρ | = | fluid density (kg/m3) | | A | = | cross-sectional area (m2) | | v | = | speed (m/s) |
| Fluid
resistance
|
|
At higher velocities, fluid friction can be modeled effectively using the drag equation (5.8), where the resistive force is proportional to velocity squared, i.e., Ff ∝ v2. Equation (5.8) is often called the hydrodynamic drag.
At low velocities, however, the resistive force is linear with velocity, or Ff = −bv. For spherical objects moving slowly through a fluid, the equation becomes Stokes’s law: | | | Ff | = | fluid frictional force (N) | | μ | = | dynamic viscosity (N s/m2) | | r | = | radius of sphere (m) | | v | = | speed of sphere (m/s) |
| Stokes’s law
|
A small, spherical object falling slowly in a thick, viscous fluid will rapidly reach a terminal velocity that follows Stokes’s law: | | | v | = | terminal velocity (m/s) | | ρs | = | density of sphere (kg/m3) | | ρf | = | density of fluid (kg/m3) | | μ | = | dynamic viscosity (N s/m2) | | g | = | gravitational acceleration (m/s2) | | r | = | radius of sphere (m) |
| Terminal velocity
from Stokes’s law |
You can see this for yourself by dropping a small sphere (such as a BB) into a thick liquid (such as transparent oil). The sphere will fall slowly yet it quickly reaches a steady, terminal speed.
|
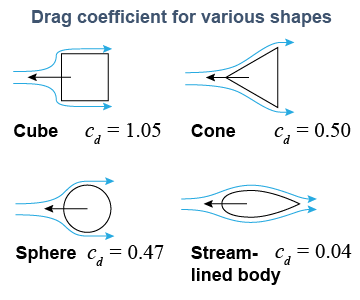Equation (5.8) gives the friction force on an object moving at speed v through a fluid of density ρ. The drag coefficient cd is a geometrical shape factor that describes the relative ease with which an object moves through air or water. An aerodynamic shape has a low drag coefficient. For example, cd is 0.04 for an airfoil. A blunt cube, in contrast, has a high drag coefficient cd = 1.05. This means that, at the same speed, the force of air resistance on a cube is 26 times greater than on an airfoil with the same cross-sectional area!
|
|
When sprinter Usain Bolt set the world record of 9.58 s for the 100 m sprint at the World Championships in 2009, his position was tracked every 0.1 s using a laser velocity guard device. Physicists published calculations using these data to show that an astonishing 92% of Bolt’s effort went into overcoming drag. They estimated his body’s drag coefficient at cd = 1.2, within the typical human range of 1.0–1.3.
|
