|
Convection is a complex phenomenon. An accurate description involves fluid flow, heat flow, friction, viscosity, surface roughness, buoyancy, and about a dozen other factors. Despite the complexity, a couple of general principles can be used to create a useful model of convection. This model applies to convective heat transfer between a surface and a fluid contacting the surface. - The rate of heat transfer is approximately proportional to the area A through which the heat flows.
- The rate of heat transfer is approximately proportional to the temperature difference ΔT between the surface and the fluid a distance away from the surface.
|
|
Equation (24.2) is widely used to describe convective heat transfer. The complicated effects of flow speed and surface conditions are grouped together in the heat transfer coefficient h. A value of h = 1 W/(m2 °C) means that one watt of heat is transferred from each square meter of area when the temperature difference is one degree Celsius. Practical heat transfer systems typically have a value of h between 10 and 10,000 W/(m2 °C).
|
| (24.2) | | | P | = | power (W) | | h | = | heat transfer coefficient (W m−2 °C−1) | | A | = | cross-sectional area (m2) | | ΔT | = | temperature difference (°C) |
| Heat convection
|
|
Equation (24.2) suggests two common ways to increase heat transfer by convection: - Increase the area A contacting the fluid and/or
- increase the heat transfer coefficient h by raising the flow rate.
|
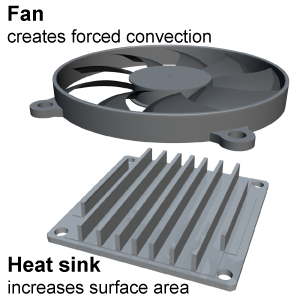Most convective heat transfer devices have fins. Fins are extensions of heat-conducting metal that can dramatically increase the surface area available for heat transfer by convection. For example, a computer chip is cemented to the finned aluminum block in the illustration. The finned surface is 20 times larger than the surface of the chip itself. The finned aluminum structure is an example of a heat exchanger. A heat exchanger is a device specifically designed to enhance heat transfer by convection.
|
|
The second part of the cooling mechanism is the fan that snaps on top of the heat exchanger. The fan forces air to flow across the surface of the fins, greatly increasing the value of the heat transfer coefficient. In practice, the use of a fan often raises the heat transfer coefficient by a factor of 10–20 over free convection.
|
|
Cooling fins and a fan can be insufficient to cool some devices, such as a room packed full of computer network servers. Such rooms are typically air conditioned, which acts to increase the temperature difference in equation (24.2) and thus to increase the convective heat transfer.
|
A finned surface increases heat transfer because it - increases the surface area contacting the fluid.
- increases the amount of metal to absorb heat.
- provides a thermal insulator between the air and the item being cooled.
- increases the temperature difference between the object being cooled and the fluid.
 |
Choice a is correct. Convection is proportional to surface area and adding fins greatly increases the surface area available to transfer heat. 
|
