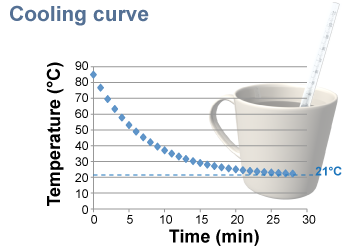|
Consider a cup of 85ºC coffee on a table in a room where the air temperature is 21ºC. Over time, the hot coffee cools down until it eventually reaches the same temperature as the air. The graph of temperature versus time, illustrated at right, is called a cooling curve. Notice that the temperature drops quickly at the start and then falls more gradually over time. This is typical of how heat is exchanged. The largest changes in temperature occur when the differences in temperature are greatest. 
|
|
Heat transfer is the study of how thermal energy, or heat, moves. The coffee cools because heat transfer moves thermal energy from the coffee and cup into the room air. In this chapter we will consider how heat moves through the mechanisms of conduction, convection, and radiation. We will also consider how quickly heat moves, which is the rate of heat transfer. Since heat is a form of energy, the heat transfer rate is energy per unit time, which has units of joules per second (otherwise known as watts).
|
|
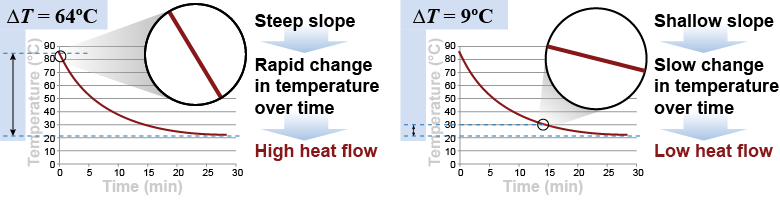
The rate of heat transfer between the coffee cup and the room depends on the difference in temperature. For example, when the temperature difference is high—such as at the start—the temperature changes by 8°C in the first minute. This represents an average heat transfer rate of 144 J/s or 144 W. Later, when the coffee has cooled to 30ºC, the temperature changes by only 1.2ºC per minute because the rate of heat transfer has dropped to 21 W.
|
|
The observation that the rate of heat transfer depends on the difference in temperature is called Newton’s law of cooling. This “law” is not really a law but a rule of thumb that applies approximately to many situations. Newton’s law of cooling makes common sense. When there is a big temperature difference, heat flows quickly and causes the temperature to change quickly, too. When there is a small temperature difference, heat flows slowly and temperature also changes slowly.
 |
You may have noticed that the cooling curve in the illustrations above has the shape of the mathematical function called the exponential. In particular, this shape is called an exponential decay and can be written as | | | T1 | = | temperature of object 1 (°C) | | T0 | = | temperature of environment (°C) | | (T1 − T0)f | = | final temperature difference (°C) | | (T1 − T0)i | = | initial temperature difference (°C) | | t | = | time (s) | | t0 | = | cooling time constant (s) |
| Newton’s law
of cooling
|
On the left-hand side of the equation is the final difference in temperature (between the object and its environment) after some amount of time t has elapsed. On the right-hand side of the equation is the initial difference in temperature. The right-hand side also includes the mathematical constant e = 2.718 raised to the power −t/t0. As time gets larger, the exponent becomes a larger and larger negative number, so e−t/t0 becomes a smaller and smaller number. In other words, as time goes on, the final temperature difference becomes smaller and smaller.
The right-hand side of the equation includes a variable called the cooling time constant t0. Large values of the cooling time constant mean that it takes a long time for the material to cool; small values of the cooling time constant correspond to fast cooling times. The cooling time constant can also be expressed in terms of other properties of the material: | | | t0 | = | cooling time constant (s) | | m | = | mass of substance (kg) | | cp | = | specific heat (J kg−1 °C−1) | | h | = | heat transfer coefficient (W m−2 °C−1) | | A | = | surface area (m2) |
| Cooling time
constant
|
The heat transfer coefficient h will be introduced later in this chapter on page 710.
We will see another example of exponential decay in radioactive decay in Chapter 27. 
|
|
When solving problems like the cooling coffee cup, we often assume that the temperature of the room stays constant even though energy is absorbed by the room’s air. This is usually an acceptable approximation because the thermal energy from the coffee cup cannot appreciably change the large room’s temperature. The room may also maintain a constant temperature because it exchanges heat with the environment around it, such as via the its central heating (and cooling) system or through the walls with the outside air.
|
Two blocks of ice at the same temperature are dropped into two cups of water at different temperatures. Which of the following statements is most accurate? - The ice cubes gain heat from the water at the same rate because they both are at the same temperature.
- The ice cube dropped into the colder water gains heat more quickly than the ice cube dropped into the warmer water.
- The ice cube dropped into the warmer water gains heat more quickly than the ice cube dropped into the colder water.
 |
Choice c is correct. The rate of heat flow depends on the temperature difference. The temperature difference between the warmer water and the ice is greater than the temperature difference between the colder water and the ice. 
|
