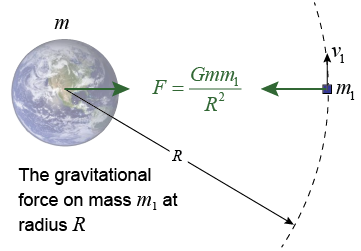Essential Physics

The orbit equation

Gravitational force and orbits

To determine whether the path of a moving object will be an orbit, consider a satellite of mass m₁, moving with linear velocity v₁, near a planet with a much larger mass m. When is the gravitational force sufficient to move the satellite in a circular orbit with radius R? To solve the problem, recall the equation for the centripetal force required to cause an object to move in a circular path of radius R. Read the text aloud

Read the text aloud

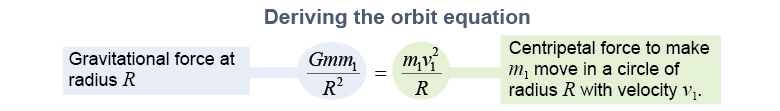
To derive the orbit equation (7.6) we set the gravitational force at radius R equal to the centripetal force needed to keep a mass m₁ moving in a circle. Read the text aloud

Read the text aloud

Deriving the orbit equation

(7.6)

R = \frac{G m}{v^{2}} v = \sqrt{\frac{G m}{R}}

R	=	orbital radius (m)
G	=	gravitational constant
	=	6.67×10^-11 N m²/kg²
m	=	mass of central object
v	=	satellite orbital speed (m/s)

Orbit equation
for circular orbits

The radius of a circular orbit depends on the mass of the planet divided by the square of the satellite’s velocity. The higher the velocity, the smaller the orbital radius. This is because it takes a stronger force to hold a faster moving object in a circular path, and gravitational force decreases with distance. A satellite with double the velocity will orbit at 1/4 the radius. As equation (7.6) shows, the velocity a satellite needs to maintain a particular orbit does not depend on the satellite’s mass, but only on the mass of the planet. Read the text aloud

Read the text aloud

Calculate the orbital velocity of a 4,000 kg satellite that orbits at a radius of 42,000 km

Calculate the orbital velocity of a 4,000 kg satellite that orbits at a radius of 42,000 km. Read the text aloud

Read the text aloud

Asked:	orbital velocity v
Given:	$R = 42,000,000 m, m = 6 \times 10^{24} kg, m_{1} = 4,000 kg$
Relationships:	v = √Gm/R
Solution:	$v = \sqrt{\frac{G m}{R}} = \sqrt{\frac{(6.67 \times 10^{- 11} {N m}^{2} / {kg}^{2}) (6 \times 10^{24} kg)}{42,000,000 m}}$ v = 3,090 m/s (6,900 mph)

Notice that the orbital velocity is quite high. The velocities are even greater at lower altitude orbits. The orbital velocity of the International Space Station is 7,700 m/s (27,700 mph) at an average altitude of 414 km above Earth’s surface. Read the text aloud

Read the text aloud

A satellite follows a circular orbit around Earth at a speed of 2,200 m/s. What is the radius of this orbit? Use m_E = mass of Earth = 5.98×10²⁴ kg.

40,000,000 m
82,400,000 m
25,600,000 m
18,200,000 m

Show

The answer is b.
Asked: radius r of the satellite
Given: Earth mass m_e = 6×10²⁴ kg, satellite velocity v = 2,200 m/s,
gravitational constant G = 6.67×10⁻¹¹ N m²/kg²
Relationships: F = Gm₁m₂/r², F_c = mv²/r
Solve:

\begin{array}{l} F = G \frac{m_{s} m_{e}}{r^{2}} \\ F_{c} = \frac{m_{s} v^{2}}{r} \end{array}

Set equations equal and rearrange to solve for r:

\begin{array}{l} r & = & G \frac{m_{e}}{v^{2}} \\ = & (6.67 \times 10^{- 11} N m^{2} / {kg}^{2}) \frac{5.98 \times 10^{24} kg}{{(2, 200 m / s)}^{2}} \\ = & 82, 400, 000 m \end{array}


216