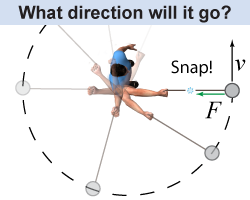|
Imagine whirling a small weight around your head on a string. If the string broke, the weight would fly off and no longer move in a circle. This is a consequence of Newton’s first law: A moving body will continue in a straight line if no net force acts on it. What direction will it travel? An object undergoing circular motion has a velocity that is tangential to the circle. If the string were to break, then the object would continue moving in the direction of its velocity—upward in the illustration. 
 |
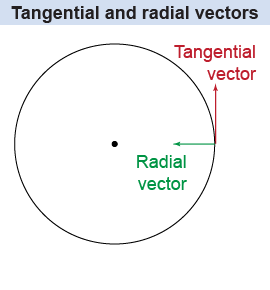To understand circular motion, it is important to distinguish between vectors that are tangential and radial. A radial vector is directed along the radius of the circle. A tangential vector is directed perpendicular to the radius at any point along the circumference of the circle. For circular motion, the radial and tangential vectors are always perpendicular to each other. In the physics of circular motion, the centripetal acceleration and force are always radial vectors, while the object’s velocity is a tangential vector. Centripetal acceleration and force are therefore always perpendicular to the velocity of an object undergoing circular motion. 
|
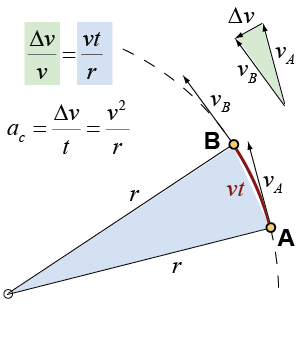What keeps the object in circular motion over your head? The string exerts an inward force that causes an inward acceleration that continually bends the object’s direction of motion toward the center of the circle. In the illustration, the velocity of the object changes direction as it moves from point A to point B. The change in velocity is represented by the vector Δv, which is perpendicular to the direction of velocity. Since acceleration is the rate at which an object’s velocity changes, the centripetal acceleration acts in the direction of the vector Δv, which is toward the center of the circle. Centripetal acceleration always points toward the center of the circle and is perpendicular to the object’s velocity.
|
|
How are acceleration and velocity related quantitatively in circular motion? Look again at the illustration above. Between points A and B the object moves a distance d = vt, while the change in its velocity is Δv. The blue- and green-shaded triangles are similar; therefore, the ratio Δv/v is the same as the ratio vt/r. Acceleration is defined as Δv/t; therefore, the centripetal acceleration is given by equation (7.3).
|
For rotational motion, it is important to distinguish among the different kinds of velocities. Linear velocity is how fast the entire wheel is moving in some direction, such as how fast a car (mounted on four wheels) is moving down the street. Angular velocity is how fast the wheel is rotating, such as in revolutions per minute (rpm). Tangential velocity is how fast an object on the circle is moving around the circle (such as a fly speck on the outer rim of a tire).
As an example, consider a truck tire with a radius of 0.5 m. The truck is moving such that the tires rotate all the way around twice every second. The tire turns by 2π radians twice every second, so its angular velocity is The car moves forward by twice the tire’s circumference every second, so the linear velocity is
The tires are not slipping on the road, which means that the tangential velocity of a speck on the outer rim of the tires (such as the tire tread) is the same as the linear velocity.
|
| (7.3) | | | ac | = | centripetal acceleration (m/s2) | | vt | = | tangential speed (m/s) | | r | = | radius of circle (m) |
| Centripetal acceleration
|
|
|
Notice from equation (7.3) that the centripetal acceleration decreases as the radius gets larger. The larger the circle, the slower the velocity has to change direction, and the lower the centripetal acceleration. The centripetal acceleration increases as the square of the velocity. That means it takes four times as great an acceleration to force an object that is moving twice as fast into the same radius of circle.
|
|
Whether a force changes a moving object’s speed or its direction depends on the force’s direction. A force in the direction of motion causes the object to change speed. A force perpendicular to the motion causes the object to change its path from a line to a circle—without changing speed. The force (or combination of forces) that points towards the center of a circle and is perpendicular to an object’s motion is called a centripetal force. Some source of centripetal force is required to keep an object in circular motion, whether it is a planet in orbit or a race car going around a curved track. We get the equation for centripetal force by combining equation (7.3) and Newton’s second law.
|
|
If you are on a rotating amusement park ride, and you feel your body being pressed outward against a wall (away from the center of rotation), are you experiencing an outwards or “centrifugal” force? For you to maintain circular motion, there must be an inward or centripetal force, as shown in the diagram above. The wall of the amusement park ride is pushing against you in an inward direction, providing this centripetal force. When people say that they are feeling a “centrifugal” force, they are referring to an apparent (or inertial) force—not a true force. Without the inward centripetal force, the inertia of your body would continue to move you in a straight line tangent to the circle, just as in the case of the mass on a string spun overhead. It is the inward centripetal force from the wall on your body that maintains your circular motion, not the sensation you have of being pushed outward against the wall.
|
| (7.4) | | | Fc | = | centripetal force (N) | | m | = | mass (kg) | | v | = | tangential speed (m/s) | | r | = | radius of circle (m) |
| Centripetal force
|
|
A Ferrari is speeding around a circular track with a radius of 400 m. At a point around the loop, it is clocked at a tangential velocity of 70 m/s. What is the Ferrari’s centripetal acceleration at this moment? - 12.25 m/s2
- 0.175 m/s2
- 24.50 m/s2
- 4.375 m/s2
 |
The answer is a: 
|
