|
The Earth orbiting the Sun, a turning wheel, and a spinning top are all examples of circular motion. The concepts of position, velocity, and acceleration used to describe linear motion take slightly different forms when used to describe circular motion. 
|
Angular velocity
|
The rate at which a rotating object spins is called its angular velocity, which is represented by the Greek letter ω (“omega”). To understand angular velocity, consider an object with a rotational position given by its angle θ. Angular velocity describes the amount that angle changes per unit time. If the angle is measured in degrees then angular velocity is expressed in degrees per second. If the angle is measured in full turns (360º), then angular velocity might be in rotations per second or cycles per second. The angular speed of motors is often given in revolutions per minute (rpm).
 |
The small electric motors used in robotics typically have rotational speeds between 8,000 and 20,000 rpm! These speeds are far too fast for typical robot motions. Gearmotors are electric motors that have small gearboxes attached. A gear ratio of 100:1 brings down the rotational speed of the output shaft to a range of 80–200 rpm, which is more suitable for moving manipulator arms and other applications. 
|
| (7.1) | | | ω | = | angular velocity (rad/s) | | Δθ | = | change in angle (rad) | | Δt | = | change in time (s) |
| Angular velocity
|
|
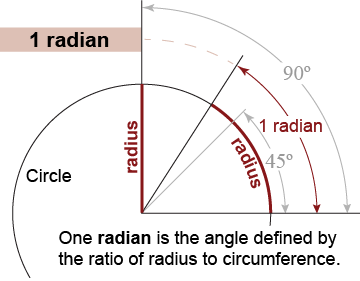For the purpose of angular speed, a radian (rad) is a more natural unit of angle than a degree. One radian equals approximately 57.3°. Radians are dimensionless because radians are a ratio of lengths. One radian is the angle formed by wrapping one radius of a circle around the circumference. There are 2π (about 6.28) radians in a full circle, which makes 2π rad = 360º.
|
|
The sign of the angular velocity depends on direction. If counterclockwise rotation is defined to be positive then clockwise rotation is negative.
|
|
Radians are pure numbers without units in the sense that meters or seconds are units. Expressed in radians per second, angular velocity has units of 1/s or s−1. If you encounter an angular velocity expressed in units of 1/s, then interpret the value as “radians per second.”
|
The Earth rotates once every 24 hours. What is its angular velocity in radians per second? | Asked: | angular velocity ω | | Given: | Earth makes one full rotation (2π rad) every 24 hours. | | Relationships: | | | Solution: | | | Answer: | 7.27×10−5 rad/s. Note that the units “radian” are not necessary, because the radian is dimensionless. |
|
If you are running around a perfectly circular pond, and you make 1/4 of a loop every minute, what is your angular velocity in radians per second? - 0.0042
- 0.026
- 0.39
- 1.0
 |
The answer is b, 0.026 (in units of radians per second). If you run 1/4 of a loop every minute, then you run π/2 radians every 60 s. The formula for angular velocity is ω = Δθ/Δt. In this case, ω = (π/2)/60 ≈ 1.57/60, or 0.026. 
|
