- Water waves with a frequency of 4.5 Hz and a wavelength of 2.0 m are traveling across a small harbor that is 200 m wide. How long does it take the waves to travel from one side of the harbor to the other?
 Blue light has a wavelength around two-thirds of the wavelength of red light. What is the frequency of blue light relative to the frequency of red light? Blue light has a wavelength around two-thirds of the wavelength of red light. What is the frequency of blue light relative to the frequency of red light?
 Red light has a wavelength of 6.56×10−7 m and a speed of 3×108 m/s. What is the frequency of red light? Red light has a wavelength of 6.56×10−7 m and a speed of 3×108 m/s. What is the frequency of red light?
 A wave has a frequency of 1.5×1013 Hz and a speed of 3×108 m/s. What is its wavelength? A wave has a frequency of 1.5×1013 Hz and a speed of 3×108 m/s. What is its wavelength?
 A radio station broadcasts at 99.5. A radio station broadcasts at 99.5.
- What physical quantity is represented by the number 99.5?
- What are the units of the number 99.5?
- What does this number mean in terms of harmonic motion?
- If the speed of the broadcast waves is 3×108 m/s, what is its wavelength?
 Radio waves broadcast at 95.3 MHz travel at a speed of 3×108 m/s. What is the wavelength of the radio waves? (Remember that one MHz is 106 Hz.) Radio waves broadcast at 95.3 MHz travel at a speed of 3×108 m/s. What is the wavelength of the radio waves? (Remember that one MHz is 106 Hz.)
 Radio waves with a wavelength of 2.95 m travel at a speed of 3×108 m/s. In megahertz, what is the frequency of the radio waves? Radio waves with a wavelength of 2.95 m travel at a speed of 3×108 m/s. In megahertz, what is the frequency of the radio waves?
 One AM radio station broadcasts at 1,350 kHz, while an FM radio station in the same city broadcasts at 89.7 MHz. All the radio waves travel at the same speed in air. One AM radio station broadcasts at 1,350 kHz, while an FM radio station in the same city broadcasts at 89.7 MHz. All the radio waves travel at the same speed in air.
- Which one broadcasts at a higher frequency?
- Which one broadcasts at a longer wavelength?
 A wave has a frequency of 5×1014 Hz and a wavelength of 6×10−7 m. A wave has a frequency of 5×1014 Hz and a wavelength of 6×10−7 m.
- What is the wave’s speed?
- How far does the wave travel in 8 minutes and 18 seconds?
 An enormous rogue wave has an amplitude of 17.0 m, a period of 14.0 s, and a wavelength of 340 m. It strikes an ocean liner, and at the instant of impact the skipper blasts a siren to alert a nearby vessel. An enormous rogue wave has an amplitude of 17.0 m, a period of 14.0 s, and a wavelength of 340 m. It strikes an ocean liner, and at the instant of impact the skipper blasts a siren to alert a nearby vessel.
- What is the speed of the rogue wave?
- The speed of sound is 343 m/s. How long do sailors on the vessel 1,000 m away have to prepare for the wave once they hear the siren?
| |
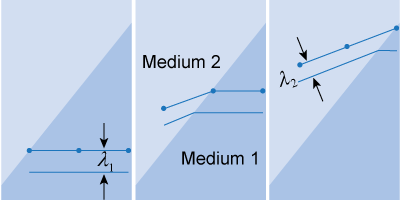
 Two parallel wavefronts are moving upward through medium 1, separated by a wavelength λ1. They impinge upon a boundary at an acute angle and refract into medium 2. There the wavefronts are separated by a smaller distance λ2. The frequency is unchanged because each wavefront must immediately pass through the boundary. Two parallel wavefronts are moving upward through medium 1, separated by a wavelength λ1. They impinge upon a boundary at an acute angle and refract into medium 2. There the wavefronts are separated by a smaller distance λ2. The frequency is unchanged because each wavefront must immediately pass through the boundary.
- In which medium are these waves faster?
- Measurements indicate that λ2/λ1 = 2/3. What is the ratio of the wave speeds, v2/v1?
- What is the ratio of the frequencies, f2/f1?
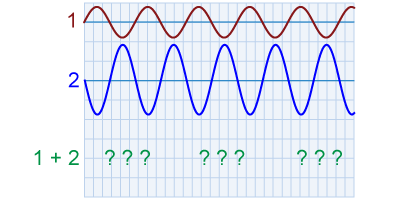
- Wave 1 and wave 2, shown here, are superposed. How will the amplitude of the sum of the two waves compare to those of the original waves? The wavelength? The phase?
- A large wave with an amplitude of 40 cm and a wavelength of 2.0 m undergoes interference with a second wave with an amplitude of 15 cm and a wavelength of 2.0 m.
- If the waves are 180° out of phase, what is the amplitude of the combined wave?
- If the waves are in phase, what is the amplitude of the combined wave?
- The “E” string on a guitar has a length of 64 cm and vibrates with a fundamental frequency of 330 Hz.
- What is the wavelength of the wave in the string?
- What is the speed of the wave in the string?
- The speed of sound in air is 343 m/s. What is the wavelength of sound produced by the vibrating string?
|

