|
When a wave reflects from a boundary the reflection is another wave that interferes with the original wave. Boundaries often create situations where the initial wave and one or more reflections produce interference effects that can be surprising. The acoustical phenomenon of reverberation is one such effect. The extraordinary tides in the Bay of Fundy are another. 
|
|
A standing wave occurs when there is constructive interference between a wave and its reflections from a boundary. You can create a standing wave by shaking one end of a spring so that wave pulses are launched at just the right rate. If the rhythm is correct, each new pulse adds constructively to the reflection of the previous pulse. This constructive interference is what causes the amplitude to grow into a standing wave.
|
|
|
Standing waves are a form of resonance. Resonance in waves occurs when boundaries reflect a wave back on itself. The resonance for a vibrating spring (or string) occurs when the length of the spring is any multiple of half a wavelength. The diagram shows a standing wave where the length of the spring equals one full wavelength. One full wavelength is a complete “S” pattern, which has three nodes and two antinodes. A node is a stationary point where the amplitude is zero. An antinode is a point of maximum amplitude. In general, resonance occurs when the size of a system is proportionally related to the wavelength of a wave.
|
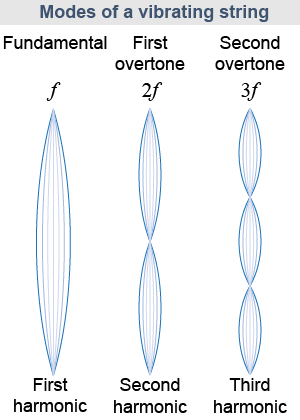At different frequencies, different standing wave patterns appear on a vibrating string. The standing wave with the lowest frequency and longest wavelength is the fundamental, which is also called the first harmonic. The next higher frequency wave is the second harmonic at twice the frequency of the fundamental. The third harmonic has three times the frequency of the fundamental, and the pattern continues. Each harmonic is a vibrational mode of the string. A mode is a characteristic pattern of vibration that occurs at a resonance frequency of a system. The frequencies at which resonant modes occur are the natural frequencies of the system. Like the vibrating string, most systems have many modes and corresponding natural frequencies.
 |
The second harmonic is also called the first overtone, and the third harmonic is also called the second overtone. Always keep track of whether you are talking about harmonics or overtones! 
|
