|
Anyone who lives near the shore knows that the tides cause the ocean level to rise and fall roughly twice a day. In Galveston Bay near Houston, the water level rises and falls about 35 cm between high tide and low tide. In Nova Scotia’s Bay of Fundy the tides are enormous, changing the water level by as much as 15 m twice a day! That is about the height of a five-story building. The tidal flows are so large that powerful whirlpools form where water flowing out from the previous tide meets the incoming tide rushing in. Why are the tides so great at this place? The answer is resonance and the explanation comes later in this section! 
|
Multiple waves
|
If you watch the ocean, you see ripples on top of waves: These are really small waves on the surface of larger waves. In most situations waves of many different amplitudes, wavelengths, and frequencies are present at the same time. The superposition principle explains how individual single-frequency waves add up to a complex many-frequency wave.
|
|
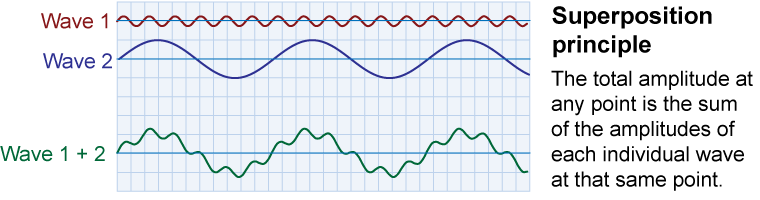
The superposition principle says that the total amplitude at any point in space and time is equal to the sum of the amplitudes of all waves that occur then and there. For example, wave 1 in the figure below has a single wavelength and frequency, as does wave 2. By adding up the independent amplitudes from wave 1 and wave 2 at the same point in space and time, we create their combined waveform, wave 1 + 2, which contains two different wavelengths and frequencies at the same time. This example shows the superposition of two waves, but in a real-world situation there may be many waves present at the same time and in the same place.
|
|
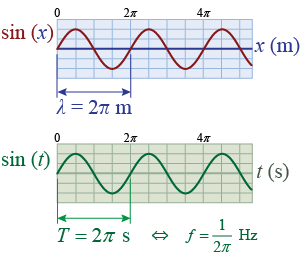The simplest wave can be completely described with a single wavelength, a single amplitude, and a single frequency. A single-frequency wave is often referred to as a sine wave because the graphs of both amplitude versus position and amplitude versus time can be described by the sine function. As an example, consider simple sine waves as a function of position x (in meters) and of time t (in seconds). The sine function repeats every 2π; therefore, sin(x) has a wavelength of 2π m. Likewise, sin(t) has a period of 2π s and a frequency of (1/2π) Hz.
|
| |
|
