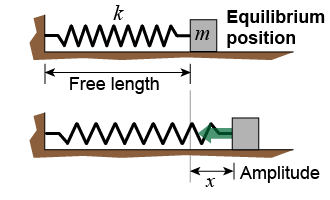Essential Physics

Natural frequency of a mass and spring oscillator


How do we analyze a spring–mass oscillator?	Consider a mass m moving on a horizontal frictionless surface. The mass is connected to a spring with spring constant k that is fixed at one end. Although the force approach could be used to determine the natural frequency of this oscillator, the energy approach provides a more direct and insightful way to treat the mass and spring.
Energy of the oscillator	At maximum amplitude the spring is extended a distance x. At this point the velocity v of the mass is zero, and the energy of the system is entirely elastic potential energy. A quarter-cycle later the mass reaches its maximum velocity v_max when the spring is at its free length and the restoring force is zero. Here, the energy of the system is entirely kinetic. Conservation of energy says that the elastic potential energy at x equals the kinetic energy at x = 0. As the mass moves back and forth the energy oscillates between elastic potential energy in the spring and kinetic energy of the moving mass.

Estimating the period and frequency	The period is the time it takes the mass to complete a full cycle. The average speed of the moving mass may be estimated as ½v_max. Since the distance traveled is 4x, we can divide this by the average speed to calculate the period. This estimated result is shown below along with the exact calculation. The estimated frequency f_est is the inverse of the period. $\begin{matrix} t = \frac{d}{v} & \to & T = \frac{4 x}{\frac{x}{2} \sqrt{\frac{k}{m}}} & \to & T_{} \approx 8 \sqrt{\frac{m}{k}} & T_{} = 2 π \sqrt{\frac{m}{k}} \\ f = \frac{1}{T_{}} & \to & f_{e s t} \approx \frac{1}{8} \sqrt{\frac{k}{m}} & f = \frac{1}{2 π} \sqrt{\frac{k}{m}} \end{matrix}$
What does this mean?	The frequency of a mass–spring oscillator depends on the square root of the ratio of the spring constant k divided by mass m. A spring that is four times stiffer (4 × k) will have twice the natural frequency. Increasing the mass by a factor of 4 reduces the frequency by a factor of 2. Note that the only difference between the exact calculation and the estimation is that the factor of 8 becomes 2π.
Test your knowledge	A mass–spring oscillator has a frequency of 10 Hz and a spring constant with a value of k = 1,000 N/m. What will the frequency of the oscillator be if the spring is replaced by one with k = 9,000 N/m?
Test your knowledge	The frequency depends on the square root of the spring constant. The spring constant increases by a factor of 9. The square root of 9 is 3, and therefore the new frequency will be three times greater, or 30 Hz.


400