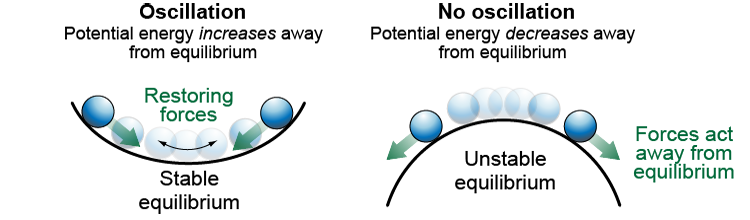
|
Harmonic motion is a characteristic of what physicists call stability. Consider the two diagrams below. The ball in the valley will oscillate when disturbed. The ball on the right will not. Both start from an equilibrium point but the stable system has restoring forces that pull the system back toward equilibrium. Oscillation occurs whenever a system has restoring forces that give it stability and act to return it to the equilibrium position. Once you know what to look for, you can predict when harmonic motion is likely to occur. 
|
|
Another way to look at a system is to consider how its potential energy changes. Any system for which the potential energy increases away from equilibrium will be stable and will oscillate. Any system for which the potential energy decreases away from equilibrium will be unstable. A system for which the potential energy does not change is neutral—neither stable nor unstable.
 |
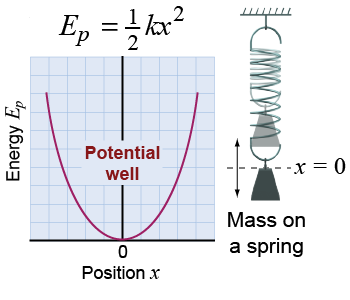Consider a mass on a spring. Let x = 0 be the equilibrium position of the mass. The equation for elastic potential energy is Ep = ½kx2. This function increases when x goes either up or down from equilibrium. The exact shape of the energy versus position graph is a parabola. The elastic potential energy increases on either side of equilibrium, and therefore the system is a stable oscillator. A potential energy graph that has a valley is called a potential well and is characteristic of stable systems that oscillate. The graphs of potential energy versus position tell a lot about how a system behaves when it is displaced from equilibrium. 
|
|
To understand the effect of inertia, think about the ball in the valley. The ball is raised up a little to one side and then released. When it reaches the bottom of the valley the net force on the ball may be zero, but its motion causes it to keep going past equilibrium. Now the restoring force pulls back, eventually reversing the motion. When the ball gets back to the center, its motion—now going in the opposite direction—causes it to overshoot again. The tendency for the ball to overshoot its equilibrium position every time is caused by its inertia. All systems that oscillate on their own have some type of restoring force, as well as some property that acts like inertia, causing the motion to overshoot the equilibrium position.
|
The concept of stability is critical to flight. Wind gusts often pitch a plane slightly up or down. Consider an aircraft with fins in the front versus one with fins in the back. If the fins are in the front, any slight pitch of the plane results in a force that causes the plane to rotate more. This is called positive feedback and it makes the plane unstable. When the fins are in the back, air flowing over the tail creates forces that push the plane back toward level flight. These restoring forces create negative feedback, reducing pitch and creating more stable flight. The farther back the tail is from a plane’s center of mass, the more stabilizing is the effect.
|
|
The trade-off for stability is maneuverability. A plane that is very stable, with tail fins far rearward from the center of mass, cannot climb or dive rapidly. Fighter planes are designed to balance aerial agility with stability. That is why fighter planes are shorter relative to their wing surface and have tail surfaces not too far behind their center of mass. These planes are twitchy to fly because they turn quickly and are buffeted by air currents far more than big passenger jets. In an aerial battle, however, these planes can change direction much more quickly.
|
_______ is the restoring force for a pendulum and _______ is the restoring force for a mass oscillating on a spring. - Centripetal force, gravitational force
- Normal force, friction force
- Air resistance, normal force
- Gravitational force, elastic force
 |
The correct answer is d. In a pendulum the restoring force is gravity, which constantly pulls the bob toward the center position.
For a mass oscillating on a spring, the spring itself, not gravity, exerts elastic force on the mass to pull it toward the center. 
|
