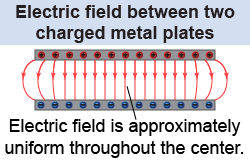|
Inside a capacitor are two metal plates that are separated by a small gap. When positive charge builds up on one plate, an equal but opposite charge builds up on the other plate. The charged plates create an electric field between them. In the center, the electric field is nearly uniform, but the field curves a bit near the edges of the plates. Charged parallel plates are used to create uniform electric fields, such as when focusing the electron beam in a cathode ray tube. 
|
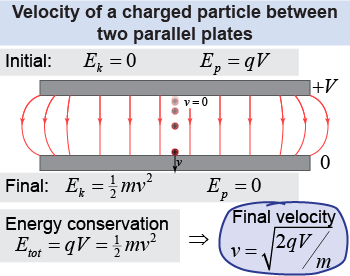The electric field created between charged parallel plates can also be used to accelerate charged particles parallel to the field direction. If a positively charged particle is set free close to the positively charged plate, how fast will it be moving when it reaches the negatively charged plate? This problem can be solved using energy conservation. Initially, the charged particle has positive electrical potential energy Ep = qV but no kinetic energy (because it starts at rest). At the end, it has kinetic energy Ek = ½mv2 but no electrical potential energy (because we set the reference potential as zero for the “negative” plate). Equating initial and final energies leads to the final velocity for the charged particle:
|
How can you calculate the capacitance for two parallel metal plates? The capacitance increases as the area of the plates is increased. The capacitance also increases as the separation between the plates is decreased; the closer the plates are to each other, the more charge the capacitor can store per volt. The capacitance for a parallel plate capacitor is given in equation (18.9).
|
| (18.9) | | | C | = | capacitance (F) | | ε | = | permittivity (C2 N−1 m−2) | | A | = | area of the plates (m2) | | d | = | separation between plates (m) |
| Capacitance
parallel plate capacitor |
|
|
In equation (18.9), a new quantity was introduced: the permittivity ε of the material between the plates. The permittivity is a measure of how much electric field is created in a material per unit charge. The permittivity of a vacuum is ε0 = 8.9×10−12 C2/(N m2). Materials with higher values of ε are used between the plates to improve the strength of the electric field and hence increase capacitance. Typical dielectrics improve the performance of capacitors by factors of 2–8.
|
